How does the demand for domestic versus foreign goods react to a change in relative prices? The answer is central to a host of policy problems in international trade and macroeconomics. To name but a few, these include the welfare effects of globalisation (Costinot and Rodriguez-Clare 2014), trade balance adjustments (Imbs and Mejean 2015), and the exchange rate pass-through of monetary policy (Auer and Schoenle 2016). In particular, any attempt to evaluate the effect of tariffs depends crucially on the assumed reaction of relative demand to relative prices (Waugh 2019, Freund et al. 2020, Larch et al. 2019).
In most models, the demand reaction is governed by the constant elasticity of substitution between domestic and foreign goods. The size of the elasticity used for calibration often drives the conclusions of the model, as shown by Schurenberg-Frosch (2015), who recomputes the results of 50 previously published models using different values for the elasticity. She finds that, with plausible changes in the elasticity, the results change qualitatively in more than half of the cases. As Hillberry and Hummels (2013) put it, “it is no exaggeration to say that [the elasticity] is the most important parameter in modern trade theory”.
Central banks and the elasticity
Elasticity is also crucial within discussions concerning unconventional monetary policy, a topic that will gain renewed urgency if the Covid-19 malaise lingers into 2021 and beyond. Consider, for example, two European central banks that, in the wake of the Great Recession, introduced exchange rate floors to limit their currencies' appreciation against the euro: the Swiss National Bank and the Czech National Bank. Currency depreciation (relative to the counterfactual without the currency floor) produces two effects relevant to the aggregate price level.
First, imported goods become more expensive, which directly increases inflation. With a large elasticity of substitution between domestic and foreign goods, however, this effect becomes muted and delayed because consumers shift toward relatively cheaper domestic goods. Second, currency depreciation stimulates the economy by encouraging exports and discouraging imports, which raises inflation in the medium term. With a larger elasticity of substitution, this effect strengthens. Because both the Swiss National Bank and the Czech National Bank use open-economy dynamic stochastic general equilibrium models for policy analysis, the assumed size of the ‘Armington elasticity’ played an important role in the decision on when and how to implement the exchange rate floor.
A meta-analysis
Despite this, no consensus on the magnitude of the elasticity exists. In different contexts, researchers tend to obtain substantially different estimates, as observed by Feenstra et al. (2018) and many other commentators before them. In new paper (Bajzik et al. 2020), we assign a pattern to these differences, a pattern that we hope will be useful for calibrating policy models in international trade and macroeconomics. As the Armington-style literature reaches its 50th anniversary, the time is ripe for taking stock. In one of the largest meta-analyses ever conducted in economics, we collect 3,524 estimates of the elasticity of substitution between domestic and foreign goods and construct 32 variables that reflect the context in which researchers produce their estimates.
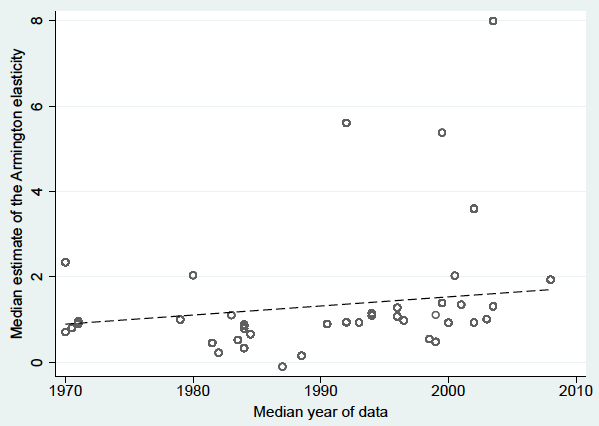
Figure 1 On average, reported elasticities increase and diverge with time
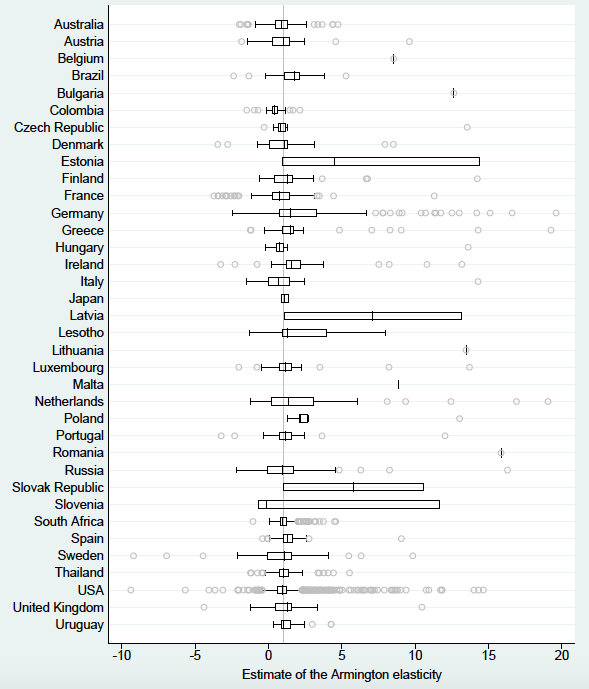
A bird's-eye view of the literature (Figure 1 and Figure 2) shows three stylised facts. First, the estimates of the elasticities vary substantially, even within individual countries. A researcher wishing to calibrate her policy model has plenty of degrees of freedom; she can easily find empirical evidence for any value of the elasticity between zero and eight. Such plausible (that is, justifiable by an empirical study) changes in the elasticity can have decisive effects on the results of the model. For example, Engler and Tervala (2016) show that changing the elasticity from three to eight more than doubles the estimated welfare gains from the Transatlantic Trade and Investment Partnership.
Second, the reported elasticity seems to be increasing in time, but it is not clear whether the apparent trend reflects fundamental changes in preferences or improved data and techniques used by more recent studies. Finally, the third stylised fact is that newer studies show more disagreement on the value of the elasticity of substitution. That is, instead of converging to a consensus value, the literature diverges.
The increased variance in the estimated elasticities provides additional rationale for a systematic evaluation of the published results. For this evaluation we use the methods of meta-analysis, which were originally developed in, or inspired by, medical research. Recent applications of meta-analysis in economics include Imai et al. (2020) on the present bias, Card et al. (2018) on the effectiveness of active labor market programmes, and Havranek and Irsova (2017) on the border effect in international trade. For recent Vox columns on meta-analysis, see, for example, Ahlfeldt and Pietrostefani (2019), Bruno et al. (2017), and Rose (2016).
Figure 2 Estimates of the elasticity vary both within and across countries
Biases in the literature
Meta-analysis allows us to correct for potential publication bias. Publication bias arises when, holding other aspects of study design constant, some results (for example, those that are statistically insignificant at standard levels or have the ‘wrong’ sign) have a lower probability of publication than other results (Stanley 2001). In the context of the elasticity of substitution, it is safe to assume that its sign is positive: a negative value is not compatible with any commonly applied model of preferences. Similarly, it is difficult to interpret a zero elasticity.
As a result, from the point of view of an individual study it makes sense not to report such unintuitive estimates, but instead to find a specification where the elasticity is positive (because non-positive elasticity suggests that something is wrong with the data or the model). Nevertheless, non-positive estimates will occur from time to time simply because of sampling error. For the same reason, researchers will sometimes obtain estimates much larger than the true value. If large estimates (which can be intuitive) are kept but non-positive ones are omitted, an upward bias arises.
Paradoxically, publication bias can improve inferences drawn from some individual studies since they avoid making central conclusions based on negative or zero elasticities. However, publication bias inevitably distorts inference drawn from the literature as a whole. Ioannidis et al. (2017) show that, in economics, the effects of publication selection are dramatic and exaggerate the mean reported estimate twofold.
To correct for publication bias, we use meta-regression techniques based on Egger et al. (1997) and their extensions, together with three new non-linear techniques developed specifically for meta-analysis in economics. The first one is due to Ioannidis et al. (2017) and relies on estimates that are adequately powered. The second technique was developed by Andrews and Kasy (2019) and employs a selection model that estimates the probability of publication for results with different ‘p-values’. The third non-linear technique is the so-called stem-based method by Furukawa (2019), a non-parametric estimator that exploits the variance-bias trade-off. All techniques suggest an upward bias in the mean reported elasticities due to publication selection.
While publication selection creates an upward bias, estimates of lower quality seem to yield a downward bias. We exploit our large dataset and the relationships unearthed by model averaging analysis of 32 variables (reflecting the context in which the estimates were obtained) to compute a mean effect corrected for publication bias but conditional on the design of the most reliable studies. In an alternative approach, we divide the estimates into groups based on the quality of the journal and the preferences of the authors themselves, while still controlling for publication bias.
Bottom line
The implied Armington elasticity corrected for the biases lies in the range 2.5-5.1, with a median estimate at 3.8. We interpret the number and the interval as our best guess on how to calibrate a model that allows for only one parameter to govern the aggregate elasticity of substitution between domestic and foreign goods (for example, an open economy dynamic stochastic general equilibrium model of the type used in many central banks). In our paper we also report implied mean elasticities for individual countries. The results are relevant to a broader discussion on the trade costs elasticity, because in a simple setting an Armington elasticity of 3.8 translates to a trade cost elasticity of 2.8 (see Costinot and Rodriguez-Clare 2014).
References
Ahlfeldt, G and E Pietrostefani (2019), “The economic effects of density: A synthesis”, VoxEU.org, 22 February.
Andrews, I and M Kasy (2019), “Identification of and correction for publication bias”, American Economic Review 109(8): 2766–2794.
Armington, P S (1969), “A theory of demand for products distinguished by place of production”, IMF Staff Papers 16(1): 159–178.
Auer, R A and R S Schoenle (2016), “Market structure and exchange rate pass-through”, Journal of International Economics 98(C): 60–77.
Bajzik, J., T. Havranek, Z. Irsova, & J. Schwarz (2020): “Estimating the Armington Elasticity: The Importance of Study Design and Publication Bias”, Journal of International Economics 127, 103383.
Bruno, R, N Campos and S Estrin (2017), “Gauging globalization”, VoxEU.org, 25 May.
Card, D, J Kluve and A Weber (2018), “What Works? A Meta Analysis of Recent Active Labor Market Program Evaluations”, Journal of the European Economic Association 16(3): 894–931.
Costinot, A and A Rodriguez-Clare (2014), “Trade Theory with Numbers: Quantifying the Consequences of Globalization”, in G Gopinath, E Helpman and K Rogoff (eds) Handbook of International Economics 4(4): 197–261.
Egger, M, G D Smith, M Schneider and C Minder (1997), “Bias in meta-analysis detected by a simple, graphical test”, British Medical Journal 315(7109): 629–634.
Engler, P and J Tervala (2018), “Welfare Effects of TTIP in a DSGE Model”, Economic Modelling 70: 230-238.
Feenstra, R C, P Luck, M Obstfeld and K N Russ (2018), “In search of the Armington elasticity”, The Review of Economics and Statistics 100(1): 135-150.
Freund, C, M Maliszewska, A Mattoo and M Ruta (2020), “The impact of the China-US trade agreement on developing countries”, VoxEU.org, 18 March.
Furukawa, C (2019), “Publication bias under aggregation frictions: Theory, evidence, and a new correction method”, working paper, MIT.
Havranek, T and Z Irsova (2017), “Do borders really slash trade? A meta-analysis”, IMF Economic Review 65(2): 365-396.
Hillberry, R H and D Hummels (2013), “Trade elasticity parameters for a Computable General Equilibrium model”, in P B Dixon and D W Jorgenson (eds) Handbook of Computable General Equilibrium Modeling 1(18): 1213-1269.
Imai, T, T A Rutter and C F Camerer (2020), “Meta-Analysis of Present-Bias Estimation Using Convex Time Budgets”, Economic Journal, forthcoming.
Imbs, J M and I Mejean (2015), “Elasticity optimism”, American Economic Journal: Macroeconomics 7(3): 43-83.
Ioannidis, J P, T D Stanley and H Doucouliagos (2017), “The Power of Bias in Economics Research”, Economic Journal 127(605): F236–F265.
Larch, M, J-A Monteiro, R Piermartini and Y Yotov (2019), “Trade effects of WTO: They're real and they're spectacular”, VoxEU.org, 20 November.
Rose (2016), “Why estimates of the trade effects of the Eurozone vary so much”, VoxEU.org, 19 October.
Schurenberg-Frosch, H (2015), “We Could Not Care Less About Armington Elasticities–But Should We? A Meta-Sensitivity Analysis of the Influence of Armington Elasticity Misspecification on Simulation Results”, Ruhr Economic Papers 594/2015, Ruhr Universitat Bochum, Germany.
Stanley, T D (2001), “Wheat from Chaff: Meta-analysis as Quantitative Literature Review”, Journal of Economic Perspectives 15(3): 131-150.
Waugh, M (2019), “The US-China trade war is harming communities in the US”, VoxEU.org, 19 November.