Editor’s note: The paper behind this column appears in the first issue of CEPR’s new initiative, Covid Economics: Vetted and Real-Time Papers
COVID-19 has now spread to all parts of the world and in response, many governments have introduced draconian mitigation measures such as travel restrictions, social distancing, and the closure of schools, restaurants, and other businesses. While it is not yet clear how effective these measures have been in slowing the epidemic, one thing is clear: the measures have caused significant economic damage. Just to give one example, according to the U.S. Employment and Training Administration, the number of jobless claims during the weeks ending on 21 and 28 March and 4 April were 3.3, 6.9, and 6.6 million, respectively – an order of magnitude larger than the previous high of 695,000 recorded in October 1982. These job losses are likely due to the strict measures that have been in place in the US since the week of 15 March. According to Baker et al. (2020), it is policy response, not the virus itself, that has created the economic calamity.
The mitigation measures are aimed at preventing the peak of the epidemic from overwhelming the healthcare system and buying time to produce necessary medical equipment such as gloves, masks, and respirators (Anderson et al. 2020). They are also influenced by the experience of the city of Wuhan, China, where COVID-19 likely originated but appears to have been contained after the lockdown of the city since 23 January.
In this column, I present my reservations about the effectiveness of such draconian mitigation policies. According to basic epidemic models, the dynamics of the epidemic are governed by the transmission rate and the recovery rate. The ratio between these two parameters is called the reproduction number, which is the average number of people to whom an infected individual transmits the disease. The larger this number, the more severe the peak but the shorter the epidemic. Available data on COVID-19 suggest a reproduction number of around 2.5-3 in the absence of intervention. Because the epidemic does not end until the number of people that recover exceeds the number of newly infected individuals, stopping the epidemic requires about 66% (one minus the reciprocal of the reproduction number, assumed to be 3) of the population to be immune. The situation where a sufficient number of people have acquired immunity to stop the epidemic is called ‘herd immunity’. In the absence of vaccines, the only way to acquire herd immunity is to wait until 66% of the population have been infected and recovered.
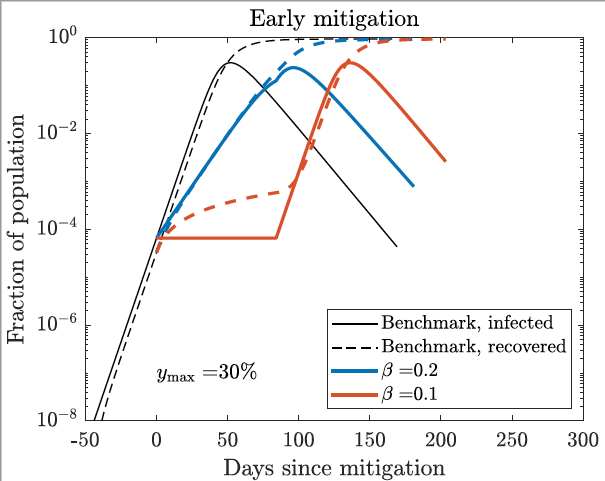
Now the dilemma is that the drastic mitigation measures that aim at reducing the peak of the epidemic also prolong the epidemic, which may cause significant economic damage. My recent paper (Toda, 2020) explores the dynamics of a standard epidemic model calibrated to observations available on COVID-19. I assume that the daily rate (recovery rate) at which an infected individual recovers (or dies) so that the person is no longer able to infect others is 0.1. The estimated daily rate (transmission rate) at which an infected individual transmits the disease to someone else is 0.3 times the fraction of the population that has not yet developed immunity. The ratio between these two parameters is the reproduction number 3. Given a fraction of the original population that is infected or immune, it is then a simple matter to predict the fraction of the population that will be infected at any given time. This is plotted as the solid black curve in Figure 1.
Figure 1
I then consider an alternative scenario in which the government takes early mitigation measures. I assume that these mitigation measures (1) are introduced when one in 10,000 of the population is infected, (2) reduce the transmission rate to 0.2 or 0.1, and (3) are lifted after 12 weeks. This scenario roughly corresponds to the situation in California, where mitigation measures were introduced around 15 March when there were about 400 cases in a population of 40 million, assuming only one in ten cases are reported. (As Gros 2020 and Stock 2020 argue, the likely significant underreporting due to the lack of random testing is further contributing to policy uncertainty.) The solid blue curve shows the number of infections for a policy that succeeds in reducing the transmission rate to 0.2, and the solid red curve for a policy that reduces the transmission rate to 0.1. We can see from the figure that the mitigation measures delay the peak of the epidemic but have almost no effect on its size, with a peak infection rate of 30%. This is because by reducing transmission, mitigation measures prevent the population from acquiring herd immunity, and the epidemic restarts as soon as the mitigation measures are lifted. To reduce the peak under this scenario, it is necessary to take mitigation measures for a far longer period than 12 weeks.
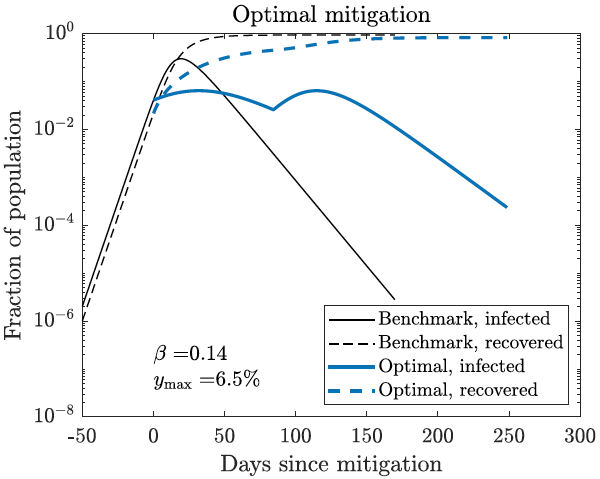
Next, let us consider how the outcome is influenced by the timing at which the mitigation measures are implemented. The assumptions here are that (1) the mitigation measures are lifted after 12 weeks, but (2) the government can choose when to implement the measures as well as target the transmission rate. Figure 2 shows the course of the epidemic under this scenario when the government chooses the timing in order to minimise the peak number of infected individuals. In this case there are two peaks: one during the period when the mitigation measures are implemented, and another after they are lifted. By starting mitigation measures only when about 1% of the population is infected and targeting a transmission rate of 0.14 (which is close to the number estimated in many countries as of early April), the peak number of infections can be reduced from 30% of the population under the baseline case to 6.5% under the optimal mitigation measures. While a peak infection rate of 6.5% may still be too large compared to the capacity of the healthcare system, it is far lower than the benchmark case of 30%. Reducing the peak may be crucial from a public health perspective since the mortality during the 1918 Spanish flu epidemic was much higher around the peak (Trilla et al. 2008).
Figure 2
Based on these simulation results, I am sceptical about the draconian lockdown measures currently being taken in many countries, for two reasons. First, according to the model, the measures need to be taken for a prolonged period perhaps far exceeding 12 weeks. That seems economically unsustainable. Second, while it is theoretically possible that we can contain the virus (i.e. stop new infections completely) by taking drastic measures, such a situation is not stable. Unless we acquire herd immunity, the epidemic will restart once a few new cases arise, taking us back to the original situation. In democratic, free market economies, having no epidemic is a knife-edge, unstable equilibrium in the absence of herd immunity.
I think it is crucial for policymakers to think about the exit strategy from lockdowns (Baldwin 2020, Ichino et al. 2020). We can live in lockdown for a few weeks – perhaps for a month or two – but we cannot be locked down forever.1 For example, according to Dingel and Neiman (2020), only one-third of jobs in the US can be done from home. Given that we cannot expect a vaccine to be developed and to pass the Phase III clinical trial any time soon, we should accept the uncomfortable truth that the epidemic will not end until about two-thirds of us have been infected, which is likely very far from the present situation. If that is the case, we need to design alternative mitigation measures to lockdown that are sustainable. One interesting case is Sweden, where the policy is close to the optimal policy suggested by my model. The Swedish government states that “it is important to implement the right measure at the right time”2 and has kept schools, restaurants, and other businesses open3 while limiting large gatherings and encouraging the elderly to isolate themselves. The idea behind this policy is probably to see the relatively low risk, young population become gradually infected and thus to achieve herd immunity with minimal economic disruptions.
Concluding remarks
To keep the peak infection rate within the capacities of healthcare systems, or to ‘flatten the curve’, governments need to take mitigation measures for a prolonged period. However, the drastic measures currently taken in many countries may not be economically sustainable. It may therefore be desirable to adopt milder but more sustainable measures such as (1) encouraging hygiene, (2) discouraging large gatherings and (3) targeted isolation of susceptible groups such as the elderly and those with underlying conditions, while (4) keeping economic life as normal as possible. It is also crucial to develop antibody testing to identify immune individuals and let them go back to their usual business.
References
Baker S, N Bloom, S J Davis, K J Kost, M C Sammon and T Viratyosin (2020), “The Unprecedented Stock Market Impact of COVID-19”, Covid Economics, Vetted and Real-Time Papers 1, 3 April.
Anderson R M, H Heersterbeek, D Klinkenberg and T D Hollingsworth TD (2020), “How Will Country-based Mitigation Measures Influence the Course of the COVID-19 Epidemic?”, Lancet.
Toda, A A (2020), “Susceptible-Infected-Recovered (SIR) Dynamics of COVID-19 and Economic Impact”, Covid Economics, Vetted and Real-Time Papers 1, 3 April.
Gros, D (2020), “Creating an EU ‘Corona Panel’: Standardised European sample tests to uncover the true spread of the coronavirus”, VoxEU.org, 28 March.
Stock, J (2020), “Data needs for shutdown policy”, VoxEU.org, 4 April.
Trilla A, G Trilla and C Dear (2008), “The 1918 “Spanish Flu” in Spain”, Clinical Infectious Diseases 5(1): 668-673.
Baldwin, R (2020), “Remobilising the workforce for ‘World War COVID’: A two-imperatives approach”, VoxEU.org, 13 April.
Ichino A, G Calzolari, A Mattozzi, A Rustichini, G Zanella and M Anelli (2020), “Transition Steps to Stop COVID-19 Without Killing the World Economy”, VoxEU.org, 25 April.
Dingel J and B Neiman (2020), “How Many Jobs Can Be Done at Home?”, Covid Economics, Vetted and Real-Time Papers 1, 3 April.
Endnotes
1 See https://www.nytimes.com/2020/04/06/opinion/coronavirus-end-social-distancing.html
2 See https://www.government.se/articles/2020/04/strategy-in-response-to-the-covid-19-pandemic/
3 See https://foreignpolicy.com/2020/03/24/sweden-coronavirus-open-for-business/