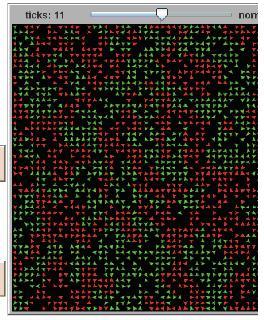
One of the (many) reasons why the Nobel laureate Thomas Schelling became famous was his analysis of sorting and segregation. He showed that differences in tastes about the kinds of people with whom one wanted to interact would lead to intensely segregated social environments. This would be true even if most people were actually rather (although not fully) tolerant about the diversity they would accept in their social medium.
Figure 1. Segregation
Figure 1 shows a typical outcome from a simulation of Schelling’s model starting from a random configuration (several applets to generate such graphs can be found in the Internet, this one is from Wilensky (1997)). Although Schelling’s study was initially motivated by urban racial segregation, there are many interesting examples of sorting in economic life. Good students tend to go to school or college with similarly good students, the most capable workers gather around similarly able people, and so on. A good reason for this was well summarised by Lucas (1988): “Human capital accumulation is a social activity, involving groups of people in a way that has no counterpart in the accumulation of physical capital.”In place of human capital accumulation in the previous quotation, of course, you can substitute production or any other meaningful activity and replace social activity with activity generating excludable externalities – it immediately suggests a model of segregation.
Such models do exist. For human capital accumulation, De Bartolome (1990) and Benabou (1993) propose models interacting local public finance and peer group (classroom) effects to create residential community segregation. For the labour market, we have, for example, Kremer and Maskin (1996) and Legros and Newman (2002).
A model of networked production
In a recent paper, we (Cabrales, Calvó-Armengol and Zenou 2008) have proposed a model where individuals make a costly production effort. Others can benefit from this productive effort by “socialising” with them, much the way academics and researchers benefit from the work of others by interacting in various forms (writing papers together, going to conferences and seminars and so on). If both the productive and the socialisation effort are costly, individuals of different ability levels will have different incentives to produce and interact with one another, and a rich pattern of interaction reminiscent of, for example, the dense network of scientific collaboration arises. An interesting feature of this model is that, in equilibrium, one can have excessive production and socialisation as well as too little activity on both fronts (with respect to a utilitarian benchmark), so that “rat-race” and “tortoise” equilibria can co-exist for the same parameter values. Also, the model predicts that an increase in the payoffs to output (or a decrease in the cost of inputs) affects more strongly the amount of socialisation than the amount of productive effort. This is an interesting result in the light of rapidly increasing scientific and R&D collaborations. But it may also explain the steady decrease in social capital building for those activities that “compete” with other, labour market-based, productive activities.
Another interesting side of this model is the rather novel incentives for segregation. As already mentioned, the model predicts that more capable people make a higher productive effort. Individuals benefit from the effort of others directly, and this is the reason why they choose to socialise with them. But there is an indirect way in which highly productive people are “useful” to others. By making a higher effort, they, in turn, increase the incentives of their connections to make effort, which then feeds back into the incentives of others, and so on. These complementarities can explain how the “rat-race” and “tortoise” equilibria appear. But the total benefit of these complementarities is larger for more highly productive individuals, who would then have larger relative payoffs in an environment of average higher productivity. This, in turn, generates the possibility of segregated equilibria, where individuals of different productivities sort into isolated groups.
To the extent that the social outcomes are not optimal in many of these models, there is a natural scope for policy. Governments understand this since they subsidise both production and also, perhaps curiously, socialisation. Funding for workshops and conferences is a routine part of support for research activities. Even the Internet, the über-socialising tool, originates from a government project to make scientific communication/socialisation easier. So the next time you get invited to an “exclusive” small workshop or larger conference, do not think of it as “leaving the ivory tower” but of contributing to its construction.
References
Cabrales, A., A. Calvó-Armengol and Y. Zenou (2008), “Social Interactions and Spillovers: Incentives, Segregation and Topology,” mimeo.
Benabou, R. (1993), “Workings of a City: Location, Education, and Production,” Quarterly Journal of Economics 108, 619-52.
De Bartolomé, C.A.M. (1990), “Equilibrium and Inefficiency in a Community Model with Peer Group Effects, ” Journal of Political Economy 98, 110-33.
Kremer, M. and E. Maskin (1996), “Wage Inequality and Segregation by Skill,” NBER Working Paper 5718.
Legros, P. and A. Newman (2002), “Monotone Matching in Perfect and Imperfect Worlds,” Review of Economic Studies 69, 925-942.
Lucas, R., Jr. (1988), "On the Mechanics of Economic Development ," Journal of Monetary Economics 22, 3-42.
Schelling, T.C. (1971), “Dynamic Models of Segregation,” Journal of Mathematical Sociology 1, 143-186.
Wilensky, U. (1997). “NetLogo Segregation model,” Center for Connected Learning and Computer-Based Modeling, Northwestern University, Evanston, IL.