There are two main benefits to a credible deposit insurance system, whether the system is national or multinational. One is that deposit insurance protects the savings of financially unsophisticated individuals and small businesses. The other major benefit relates to the short-term, demandable nature of deposits that makes them convenient for settling transactions but can also lead to a ‘bank run’. By removing the incentive for bank runs, deposit insurance can reduce the severity of financial crises and enhance financial and monetary stability.
Relative to a national system, a multinational deposit insurance system can have the added benefit of improving the credibility of deposit insurance.1 Especially in a monetary union, a purely national deposit insurance scheme could be exposed to the ‘sovereign-bank doom loop’ whereby a decline in the creditworthiness of a nation’s banking system that increases the cost of resolving bank failures impairs the government’s creditworthiness. In turn, this decline in government creditworthiness causes a loss of confidence in deposit insurance that leads to bank runs and further bank losses.2 A multinational deposit insurance system can help break this loop by sharing the losses from insuring deposits among nations.
In our work, we focus on understanding the importance of fair market value-based pricing of a multinational deposit insurance system such as the European Deposit Insurance Scheme (EDIS). We argue that if banks are not charged a premium equal to the fair cost of the deposit insurance, then various moral hazard-related distortions arise. Moreover, subsidised rates may be a source of member nation conflicts that can lower the likelihood of acceptance of the common scheme (Jokivuolle and Pennacchi 2019).
The cost of deposit insurance
Deposit insurance differs from many other types of insurance in that the risks of insuring banks’ deposits cannot be easily diversified. The risk of a deposit insurance claim from a bank failure is highly systematic. The deposit insurer’s losses tend to be low during macroeconomic expansions and high during macroeconomic contractions. As an example, Figure 1 shows the number of bank failures in the US each year since federal deposit insurance was implemented in 1934. Clearly, in most years the number of bank failures was very low, but a large proportion of failures clustered in three well-known crisis periods (the Great Depression of the 1930s, the Savings and Loan Crisis of the 1980s and early 1990s, and the Great Recession of 2008-2012).
Figure 1 Annual number of US bank failures, 1934 to 2018
Source: US Federal Deposit Insurance Corporation
Consequently, the fair market cost of deposit insurance will exceed the ‘actuarially fair’ expected loss due to the addition of a systematic risk premium needed to compensate the insurer for bearing undiversifiable risk.3
Specifically, define EDF to be a bank’s annual expected default frequency and LGD as the deposit insurer’s loss given default. Then EDF×LGD is the insurer’s annual expected losses from insuring a bank, which also equals the actuarially fair insurance premium. But the ‘fair market’ cost of providing deposit insurance, equal to the insurance premium that a bank would pay for covering this cost, is:
Fair-Market Deposit Insurance Premium = EDF×LGD + SRP
where SRP is the insurance’s systematic risk premium.
While deposit insurance differs from many other types of insurance, it is closely related to some common financial contracts. A prime example is uninsured debt that is subject to default risk, such as a corporate loan, bond, or even an uninsured bank deposit or bond. Since insurance against a debt’s default would make it default-free, this logic implies the valuation equation:
Value of Default-Risky Debt = Value of Default-Free Debt – Value of Default Insurance
Since the value of default-risky debt is less than the value of default-free debt that promises the same future payments, its lower price is reflected in a higher promised yield to maturity compared to the yield on equivalent default-free debt. The difference in these yields is referred to as the default-risky debt’s ‘credit spread’. Importantly, the value of this credit spread is analogous to a fair-market annual deposit insurance premium – both represent compensation for default risk. Consequently, theory implies that a default risky debt’s credit spread should also equal EDFLGD + SRP (e.g. Duffie and Singleton 1999).
Even more closely related to deposit insurance is another financial contract that directly insures against default losses, namely, a credit default swap (CDS) contract. The CDS spread on a firm’s debt equals the annual insurance premium that the insured (protection buyer) pays to the insurer (protection seller) to cover losses if the debt defaults. Thus, as with the debt’s credit spread, theory predicts that the fair CDS spread equals the debt’s expected default losses plus a systematic risk premium, EDF×LGD + SRP.
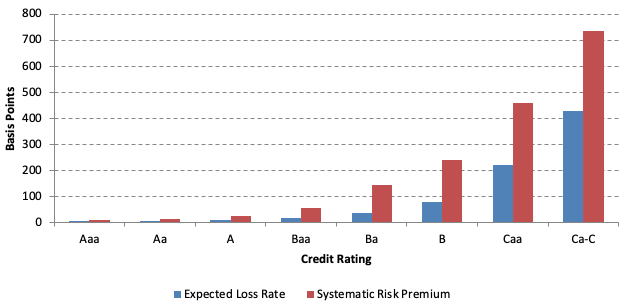
Empirical evidence strongly supports this theoretical prediction. Moreover, the size of the systematic risk premium, SRP, is substantial and typically exceeds expected losses, EDF×LGD. Figure 2 shows a decomposition for CDS spreads taken from Table III in Berndt et al. (2018). They proxy firms’ expected default losses from estimates of EDF by Moody’s Analytics and estimates of LGD from Markit, where the systematic risk premium equals the CDS residual after expected losses (their sample covers more than 500 firms over the period 2002 to 2015). The figure shows that for each credit rating, the average systematic risk premium always exceeds the average expected default loss, and the overall ratio of the systematic risk premium to expected losses is 2.92. Empirical studies that estimate the fair cost of deposit insurance premiums from bank stock market and financial statement data find similar ratios of systematic risk to expected losses on the order of 1 to 3.4
Figure 2 Expected loss rates and systematic risk premiums from credit default swap spreads
Source: Berndt et al. (2018), Table III.
In summary, fair insurance premiums incorporate a sizeable systematic risk premium that tends to increase with a bank’s expected default losses. Unfortunately, few, if any, national deposit guarantee schemes set premiums in this manner. The consequence is several moral hazard incentives. First, banks will have an incentive to prefer insured deposits. Second, banks will have an incentive to invest in securities (especially structured financial securities) and loans with excessive systematic risk because they are not charged for taking this type of risk (Pennacchi 2006, Coval et al. 2009). Empirical evidence supports this incentive for systematic risk-taking (Iannotta et al. 2019, Efing 2015). The danger is that banks will herd into systematically-risky investments that are highly likely to suffer losses during economic downturns, increasing the likelihood of systemic failures.
The European Deposit Insurance Scheme
We now consider the implications of these arguments for the proposed EDIS.5 Along with the Single Supervisory Mechanism (SSM) and the Single Resolution Mechanism (SRM), the EDIS is envisioned as the third pillar of the banking union in the EU. Transitioning from member nations’ deposit guarantee schemes (DGS) to the EDIS is planned to take seven years, during which time banks and national deposit insurance funds would contribute to the EDIS deposit insurance fund (DIF).6 After the transition, the EDIS would provide full insurance on the covered deposits of the banks of member nations, with insurance claims being paid out of the DIF and banks’ insurance premiums (contributions) being paid into the DIF. A fiscal backstop for the DIF might be provided in the form of a revolving credit line, say, from the European Stability Mechanism (ESM). While banks’ premiums will be risk-based, they will also be set to maintain the DIF’s funds at a target level equal to 0.8% of aggregate covered deposits.
Setting premiums to target a ratio of DIF funds to covered deposits is a common practice amongst deposit insurance systems, including the US Federal Deposit Insurance Corporation (FDIC), which now has a long run target for its DIF of 2% of insured deposits. Unfortunately, this practice conflicts with setting premiums that are truly risk-based (Feldman 1998, Pennacchi 2000). Rather, it makes premiums countercyclical. The reason for this is that during economic downturns when bank failures rise and the DIF is depleted, the average level of premiums must be raised to bring the DIF back to its target. Conversely, during economic expansions the DIF grows and tends to rise above its target, which leads to a cut in the average level of premiums. It is easy to see that another form of moral hazard can result because banks’ cost of insured deposits is more (less) heavily subsidised during expansions (contractions), creating an incentive to grow faster (slower) and exacerbating the credit cycle.
The proposed EDIS plans to set risk-based premiums where banks that are estimated to be riskier will pay relatively higher premiums compared to banks deemed to be safer. But during any point in the financial cycle premiums are unlikely to be risk-based in an absolute sense of fairly reflecting the cost of insurance because the need to target DIF funds forces the average premium to be countercyclical.7 Now it may be that, through the financial cycle, average premiums will approximately equal average losses to the DIF. But as argued earlier, this implies that the average insurance premium will be subsidised because it fails to include a systematic risk premium. Indeed, if banks’ insurance premiums were set fairly in a market value sense, the ratio of DIF funds to covered deposits should be expected to grow without bound due to the presence of the systematic risk premium that makes the average premium exceed the DIF’s average loss.8
One might argue that risk-based premiums based on banks’ expected losses can, at the least, prevent cross-subsidisation whereby riskier banks will not be subsidised by safer ones (Carmassi et al. 2018). However, such risk-based premiums would still represent cross-subsidisation because on a market value basis, the difference between riskier banks’ premiums versus safer banks’ premiums should be substantially greater than the difference in their expected losses (Figure 2).
This market value cross-subsidisation at the bank level may be a source of conflict in establishing the EDIS. Participation in the EDIS may result in relatively safer national banking systems providing net market value subsidies to relatively riskier national banking systems. Consequently, there has been some resistance to the EDIS or proposals that would retain national DGSs with the EDIS mainly providing a backstop to national DGSs (e.g. Bénassy-Quéré et al. 2018).
Measures to reducing distortions and conflicts
Several design features could reduce the distortions that arise from cross-bank subsidies.
- Require substantial ‘bail-inable’ equity and debt. If banks are required to have a substantial amount of liabilities that are junior (subordinated) to deposits, then deposits can be made essentially risk-free and deposit insurance becomes largely irrelevant. The credit spreads on these bail-inable junior liabilities of banks will function as fair-market insurance premiums. As a result, moral hazard distortions and deposit insurance subsidies will be mitigated. Of course, such requirements will only be effective in preventing EDIS losses if bank supervisors take prompt action to close an insolvent bank prior to losses exceeding its bail-in liabilities.
- Charge banks for the ESM’s credit line. If the ESM were to provide a backstop in the form of a credit line to the DIF, the ESM would absorb much of this systematic risk. Consequently, there is economic justification for compensating the ESM directly in the form of systematic risk premiums paid by banks. Requiring that banks pay this charge would reduce EDIS moral hazard incentives and cross-subsidies among banks. There could even be an increased agreement for the ESM to serve as a backstop.
- Allow nonbanks to share the risk of DIF targeting. There are several ways that nonbank investors can absorb the risks of managing the DIF’s level near a target (see the proposals of Kane 2003 and Pennacchi 2010). Since private investors would require compensation from the DIF that covers not only their expected losses but also a systematic risk premium, the EDIS would have economically observable justification for setting banks’ insurance premiums that cover both expected losses and these losses’ systematic risk premiums.
Conclusion
Enhancing the credibility of deposit insurance to avoid a ‘sovereign-bank doom loop’ is a clear benefit of a multinational deposit insurance system, such as the proposed EDIS. Yet some member nations may object if they fear their national banking systems will subsidise those of others. Due to the common practice of setting banks’ insurance premiums to target deposit insurance funds, these fears may be justified. Yet, we have argued that the EDIS can be designed to significantly reduce subsidies, and an added benefit is that moral hazard distortions are mitigated. These design features include a requirement for substantial bail-inable equity and debt, establishing a systematic risk charge paid by banks to the ESM for its line of credit, and managing the risk of DIF funds by involving non-banks in risk sharing.
References
Bénassy-Quéré, A, M Brunnermeier, H Enderlein, E Farhi, M Fratzscher, C Fuest, P Gourinchas, P Martin, J Pisani-Ferry, H Rey, I Schnabel, N Véron, B Weder di Mauro, and J Zettelmeyer (2018), “Reconciling Risk Sharing with Market Discipline: A Constructive Approach to Euro Area Reform” CEPR Policy Insight No 91.
Berndt, A, R Douglas, D Duffie, and M Ferguson (2018), “Corporate Credit Risk Premia”, Review of Finance 22, 419-454.
Carmassi, J, S Dobkowitz, J Evrard, L Parisi, A Silva, and M Wedow (2018), “Completing the Banking Union with a European Deposit Insurance Scheme: Who is Afraid of Cross-Subsidization?”, Occasional Paper Series No 208, European Central Bank.
Coval, J, J Jurek, and E Stafford (2009), “Economic Catastrophe Bonds”, American Economic Review 99, 628-666.
Duffie, D, R Jarrow, A Purnanandam, and W Yang (2003), “Market Pricing of Deposit Insurance”, Journal of Financial Services Research 24, 93-119.
Duffie, D, and K Singleton (1999), “Modeling Term Structures of Defaultable Bonds”, Review of Financial Studies 12, 687-720.
Efing, M (2015), “Arbitraging the Basel Securitization Framework: Evidence from German ABS Investment”, Deutsche Bundesbank Discussion Paper No. 40/2015.
Feldman, R (1998), “When Should the FDIC Act Like a Private Insurance Company? When It Comes to Pricing, Not Reserves”, Federal Reserve Bank of Minneapolis, The Region 12(3), 43-50.
Grubel, H (1979), “A Proposal for the Establishment of an International Deposit Insurance Corporation”, Essays in International Finance Vol. 133, International Finance Section, Princeton University, Princeton, NJ.
Iannotta, G, G Pennacchi, and J Santos (2019), “Ratings-Based Regulation and Systematic Risk Incentives”, Review of Financial Studies 32, 1374-1415.
Jokivuolle, E, and G Pennacchi (2019), “Designing a Multinational Deposit Insurance System: Implications for the European Deposit Insurance Scheme”, Ifo DICE report, Vol. 17, No. 1, Spring 2019.
Kane, E (2003), “What Kind of Multinational Deposit-Insurance Arrangements Might Best Enhance World Welfare?”, Pacific-Basin Finance Journal 11, 413-428.
Pennacchi, G (2000), “The Effects of Setting Deposit Insurance Premiums to Target Insurance Fund Reserves”, Journal of Financial Services Research 17, 153-180.
Pennacchi, G (2005), “Risk-Based Capital Standards, Deposit Insurance, and Procyclicality”, Journal of Financial Intermediation 14, 432-465.
Pennacchi, G (2006), “Deposit Insurance, Bank Regulation, and Financial System Risks”, Journal of Monetary Economics 53, 1-30.
Pennacchi, G (2010), “Deposit Insurance Reform” in J Brown (ed.), Public Insurance and Private Markets, AEI Press.
Endnotes
[1] As far as we are aware, Grubel (1979) is the first proposal for a multinational deposit insurance corporation. He argues that an international deposit insurer could resolve the failure of a multinational bank more efficiently.
[2] This feedback mechanism is worsened if banks have large investments in the government’s debt.
[3] The logic can be seen from the basic Capital Asset Pricing Model (CAPM). The insurer incurs gains due to premiums exceeding insurance claims when market returns are high and few banks fail. The insurer incurs losses due to insurance claims exceeding premiums when market returns are low and many banks fail. This positive ‘beta’ position of the insurer requires positive expected returns, implying premiums must exceed average insurance claims.
[4] See Pennacchi (2000, 2005) whose estimates are based on a structural model and Duffie et al. (2003) whose estimates are based on a reduced form model.
[5] The European Commission’s proposal of 24 November 2015 is available at https://ec.europa.eu/info/publications/commission-proposal-european-deposit-insurance-scheme-edis_en and amendments to the proposal have been issued in 2017 (http://ec.europa.eu/finance/docs/law/171011-communication-banking-union_en.pdf).
[6] During the first 3 years (reinsurance stage), the EDIS would provide liquidity support to national DGS and cover limited losses that exceed national deposit insurance funds. During the second 4 years (co-insurance stage), deposit insurance losses would be shared between the EDIS and national DGSs.
[7] As an example, the US FDIC implemented risk-based premiums in 1993, but since its DIF was above target from 1996 to 2006, each year over 90% of all banks were charged a zero insurance premium during this decade.
[8] See Pennacchi (2000) for a proof. In principle, cumulative DIF funds in excess of a target could be paid out as ‘dividends’ to governments in order to prevent the DIF from growing without bound. However, evidence from the U.S. is that the banking industry has succeeded in resisting this by arguing that excess past premiums should be ‘rebated’ back to them.