The recent financial crisis triggered a paradigm shift in banking regulation from a foremost microprudential approach that looks at the capital adequacy of individual institutions to a macroprudential approach that focuses on the resilience of the financial system as a whole. From the latter perspective, banks are considered not as isolated business entities, but as interacting institutions whose failure may produce externalities and put the system's stability at risk. This risk is often referred to as systemic risk. The notion of systemic risk used in this column reflects potentially large-scale losses that the banks’ debt holders (other banks, depositors, deposit guarantee schemes or investors) might suffer when a systemic event occurs.
Since the crisis struck, policy setters have been busy developing new regulatory measures for addressing both dimensions of systemic risk (see Borio 2009):
- The ‘cross-sectional dimension’ that relates to the distribution of aggregate risk in a financial system at a given point in time.
The corresponding policy issue consists in the calibration of prudential instruments according to the banks' contributions to systemic risk.
- The ‘time dimension’ that covers the evolution of aggregate risk over time;
The corresponding policy issue is to encourage banks to build up capital buffers during good times which they can draw down when times are bad.
Prominent examples of relevant regulatory instruments are the capital surcharge for global systemically important banks laid down in BCBS (2011) and the countercyclical capital buffer envisaged in the Basel III package. Each of these instruments covers one dimension of systemic risk.
Although this column focuses more on the systemically important banks’ contribution to systemic risk, the method proposed here for the calculation of systemically important banks’ capital surcharges allows them to be designed in a manner that mitigates cyclical effects. Thus, the column addresses both dimensions of systemic risk, as it affects systemically important banks, in a single integrated approach.
Relevant work and literature
Concerning systemically important banks’ contribution to systemic risk, BCBS (2011) elaborates an indicator-based methodology complemented by supervisory judgement. The selected balance sheet and other indicators reflect five categories of systemic importance – the size of banks, their interconnectedness and global activity, their substitutability and complexity. Equally weighted, the five categories form a score value on the basis on which global systemically important banks can be shortlisted and grouped into four buckets of increasing systemic importance. The bucket corresponds to (an arbitrary set of) additional loss absorbency requirements of 1, 1.5, 2 and 2.5% of risk-weighted assets. An extra bucket with a 3.5% capital surcharge is envisaged to penalise banks that become increasingly systemically important.
Although the Basel rules text suggests an indicator-based assessment methodology, it acknowledges that “[a]nother option would be to develop a model-based approach which uses quantitative models to estimate individual banks’ contributions to systemic risk”. An advantage of the model-based assessment over the Basel methodology would be that it offers a continuous measure of banks’ systemic importance which could be directly linked to the level of additionally required capital.
Among the model-based approaches for the measurement of individual banks’ contributions to systemic risk, the most widely discussed ones are the Delta-CoVaR of Adrian and Brunnermeier (2011), the MES of Acharya et al. (2012) and the Shapley value approach of Tarashev et al. (2010). These approaches can be summarised in simple terms as follows:
- Adrian and Brunnermeier rely on an empirical approach based mostly on the stock returns of the banks under consideration;
The systems’ vulnerability is described by the movement of the aggregated stock return of all banks in the sample. The authors define the risk contribution of an individual bank – Delta-CoVaR – as the amount by which the tail risk of the system increases when this bank’s stock return plummets. The authors argue that predicted values of the Delta-CoVaR based on regression analysis can be used for the calibration of the systemic risk capital surcharges designed in a countercyclical manner.
- Acharya et al. also make use of banks’ stock returns;
They quantify the systemic impact of a bank by the average return on its stock during the 5% worst days for the market. They call this measure of systemic importance the “marginal expected shortfall”, or MES. The authors embed their risk measure into an economic model in order to determine an optimal taxation policy for systemic risk.
- Tarashev et al. adopt a portfolio approach in order to assess the tail risk of a banking system in the first step;
Like a bank’s credit portfolio might incur unexpected losses, a banking system portfolio comprised of liabilities of individual banks would incur losses if one or more banks were to fail. The tail risk of this portfolio is decomposed into the banks’ individual contributions in the second step. For this purpose the authors use a game-theoretical concept called ‘Shapley value’ which states that a bank’s contribution to the risk of the portfolio is equivalent to its average contribution to the risk of all possible sub-portfolios that contain this bank. Additionally, the authors demonstrate a number of stylised examples for the calibration of macroprudential capital rules.
The application of the first two approaches for the purposes of macroprudential capital regulation has the following drawback. The underlying assumption that the capital shortfall, captured by decreasing market capitalisation, is a reliable proxy for the negative externality produced by a bank implies that this externality consists in the cost of the recapitalisation or bailout. That means that the implicit government guarantee, taken as granted until credible resolution regimes are implemented, will continue to be an acceptable option for taxpayers. This would provide the wrong incentives for systemically important banks. Moreover, Löffler and Raupach (2013) show that the CoVaR approach may set an additional wrong incentive for a bank that can lower its Delta-CoVaR by increasing its idiosyncratic risks given an unchanged interaction with the market.
In contrast, the portfolio approach chosen by Tarashev et al. implies that the negative externality of systemic risk is defined as the expected loss suffered by the banks’ debt holders when a systemic event occurs. This negative externality reflects the tail risk originating from the banking system. Unlike the other two approaches, the credit portfolio model provides a direct link between risk factors such as the banks’ size, their individual probability of default and the banks’ asset correlation and the notion of banks’ systemic importance. Therefore, bank-specific capital surcharges based on their systemic importance would incentivise the banks to reduce their systemic importance by controlling their size and correlation with other banks.
Introduction to the proposed measures of systemic risk and systemic importance
Our approach is related both to Tarashev et al. (2010) and Acharya et al. (2012). It follows Tarashev et al. (2010) as it focuses on the losses suffered by debt holders and as it is based on a credit portfolio model. It improves upon the single-factor portfolio approach used in that paper by enriching the structure of correlation links between the banks. It introduces ‘multiple systematic factors’ that govern the correlation among the banks (and, thus, the tendency to fail simultaneously). The multi-factor correlation structure allows for a differentiated treatment of different groups of banks, which can be defined, for instance, in accordance with the geographical region in which a bank is incorporated. Banks incorporated in the same region show a higher correlation with each other than with banks from other regions. The inter-regional correlation might be estimated using banking sector indices. Such estimations reveal substantial differences between geographical regions, which supports the choice of a multi-factor rather than a single-factor model. For example, the correlation between the bank indices in Africa and Japan is as low as 32%, whereas the correlation between the bank indices in Europe and North America is as high as 80%.
Instead of the Shapley value method, which is only computationally feasible for small, homogenous banking systems, we suggest using marginal contributions in order to decompose the overall systemic risk into the risk contributions of individual banks. This idea is conceptually similar to Acharya et al. (2012) but instead of a simple rule to approximate an expected shortfall from a time series of stock returns, our marginal contributions are obtained by taking derivatives of the tail risk of the system with respect to the banks’ share in the total liabilities of the system. The banks’ risk contributions can be obtained by simulation and, thus, remain feasible irrespective of the banking system’s size and structure. Intuitively, the notion of systemic importance based on marginal risk contributions relates to the expected loss to the system originating from a given bank in a situation where the system as a whole experiences large losses. Further technical details on the model description are provided in the Annex.
Systemic risk capital surcharge and countercyclical capital add-on
Based on the banks’ risk contributions, bank-specific systemic risk capital surcharges could be calculated as described in the following. The basic idea is that there should be enough capital in the system to withstand the losses which may materialise during a systemic event. This could be achieved by setting the banks’ total capital requirements in accordance with their systemic risk contributions. However, it may be the case that due, for instance, to low correlation, a bank’s risk contribution falls short of the microprudential minimum capital requirements (which are stand-alone/not system-dependent and subject to a different assessment methodology). Therefore, this we suggest determining a continuous, bank-specific systemic capital charge defined as the difference between the systemic risk contribution and the minimum required capital if this difference is positive (and zero otherwise).
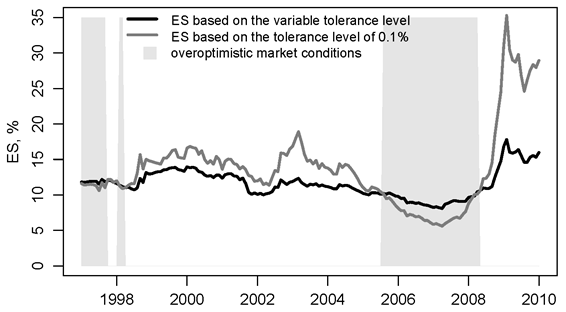
Finally, we suggest a method for calculating a capital add-on to be imposed on systemically important banks during ‘good times’ such as to mitigate cyclical effects. The starting point is the observation that market information-based estimates for the probabilities of default tend to be exceptionally low when volatility in the market decreases materially. During such calm periods, investors usually become ‘overoptimistic’ and accumulate large amounts of risk. The banks’ probabilities of default are among the input parameters of the model suggested in this column. They influence the systemic risk measure procyclically. To counteract this effect, regulators should adjust their tail-risk tolerance level over time. Suppose the ‘normal’ level of the risk tolerance corresponds to the implied probability of a systemic event equal to 0.1%. Regulators could calculate the time-varying tolerance level based on the weighted average of the estimated default probabilities for the banks under consideration. Should the time-varying tolerance level fall short of the ‘normal’ one, this would be indicative of ‘overoptimistic’ market conditions – time to introduce a countercyclical capital add-on. The bank-specific capital add-ons could then be calculated based on the difference between the individual contributions to systemic risk calculated for the time-varying tolerance level and those calculated for the normal tolerance level.
In place of a conclusion, consider the application of the suggested method to a sample of up to 86 major commercial banks from 26 countries in 1997–2010. Based on the results of this empirical study, not only could the list of global systemically important banks, identified in accordance with BCBS (2011), be closely matched – it was also possible to analyse the impact of different risk drivers on the banks’ systemic importance with regard to both the cross-sectional distribution and evolution over time.
Figure 1. Evolution of systemic risk
Annex: Model description
From the operational perspective, systemic risk is defined as the tail risk of the portfolio of the banking sector's liabilities. Starting from this definition, a widely used credit portfolio model is utilised in order to measure systemic risk and the Euler allocation principle is applied in order to decompose the system-wide risk into the additive contributions of individual institutions.
The banking sector is modelled as a portfolio of n assets, the assets representing individual banks. This portfolio can experience losses if one or more banks fail. A bank’s failure is defined within the meaning of the Merton-type structural credit risk framework: it occurs when the bank’s asset return falls below the default threshold at the risk horizon set to one year. This default threshold is specified by means of the bank’s unconditional probability of default. Exposure at risk equals the book value of the bank’s liabilities net of capital. Supposing a bank fails, its debt holders will experience a loss equal to a fraction of the exposure at risk. This is loss given default. In general, loss given default depends on the quality of the bank’s assets as well as on the level of distress in the banking system. The effect of asset fire sales and contagious losses could enter the model through this parameter. Banks are not independent. Their failures are correlated because their assets are influenced by a set of systematic factors, as described in Pykhtin (2004). A multi-factor correlation model allows, for instance, for higher correlations within specified geographical regions and lower correlation between the regions.
The probability distribution of portfolio losses at the risk horizon arises from a repeated simulation of the normally distributed systematic and idiosyncratic risk factors. This loss distribution describes completely the risk that the banking system under consideration poses to the banks’ debt holders. Specifically, the expected shortfall of the loss distribution ESq computed at a probability level q quantifies the system-wide tail risk as defined in this column. In this context q (set here to 0.999) reflects the regulator’s tolerance towards the probability of a systemic event (given as 100*(1-q) =0.1%). The ES represents the expected loss for a given portfolio in the worst 100*(1-q) per cent of cases.
Marginal risk contributions of individual banks are obtained as derivatives of the ES with respect to each bank’s share in the total liabilities of the system (i.e. portfolio weights). Marginal risk contributions multiplied by the portfolio weights result in the banks’ additive contributions to the system-wide risk. To facilitate simulation of the loss distribution and obtain robust estimates for ES and ES contributions, a variance-reducing technique is applied – the importance sampling algorithm proposed by Glasserman (2006).
Disclaimer: The views expressed here are those of the authors and do not necessarily represent those of the institutions with which they are affiliated.
References
Acharya, V, Pedersen, L, Philippon, T, Richardson, M (2012), "Measuring systemic risk“, CEPR Discussion Paper 8824, February.
Adrian, T, Brunnermeier, M (2011), "CoVaR“, Working paper 17454, NBER.
BCBS (2011), "Global systemically important banks: Assessment methodology and the additional loss absorbency requirement“, rules text, BIS.
Borio, C (2009), "Implementing the macroprudential approach to financial regulation and supervision“, Financial Stability Review No 13, Banque de France.
Glasserman, P (2006), "Measuring marginal risk contributions in credit portfolios“, Journal of Computational Finance 9, 1–41.
Löffler, G, Raupach, P (2013), "Robustness and informativeness of systemic risk“, Measures, Deutsche Bundesbank, Discussion paper series 2, No 04/2013.
Pykhtin, M (2004), "Multi-factor adjustment“, Risk Magazine 17, 85–90.
Tarashev, N, Borio, C, Tsatsaronis, K (2010), “Attributing systemic risk to individual institutions“, Working paper 308, BIS.