Exchange rates are particularly difficult to explain, and their behaviour is often at odds with existing theories. The academic literature has listed a number of ‘puzzles’ in exchange rate behaviour. For example, the famous forward premium puzzle (or Fama puzzle) implies that excess returns on foreign currency investments can be predicted by short-term interest rate differentials (and that uncovered interest rate parity, or UIP, does not hold). Froot and Thaler (1990) suggested that gradual portfolio adjustment could solve this puzzle. They argue that “at least some investors are slow in responding to changes in the interest differential”, and that “it may be that these investors need some time to think about trades before executing them, or that they simply cannot respond quickly to recent information”. In Bacchetta and van Wincoop (2010), we took this proposal seriously and showed that it can indeed account for the forward discount puzzle.
Can delayed portfolio adjustment explain other exchange rate puzzles? In a new paper (Bacchetta and van Wincoop 2019), we show that it can account for as many as six puzzles that have been identified in the literature. We propose a tractable model where results can be derived theoretically and provide numerical illustrations. To model gradual portfolio adjustment, we assume that investors face a quadratic adjustment cost of changing the international allocation of their portfolios. This implies that optimal portfolios are persistent. More precisely, the optimal portfolio today is a linear combination of yesterday’s portfolio and the portfolio that would be chosen without the adjustment cost.
Delayed exchange rate response
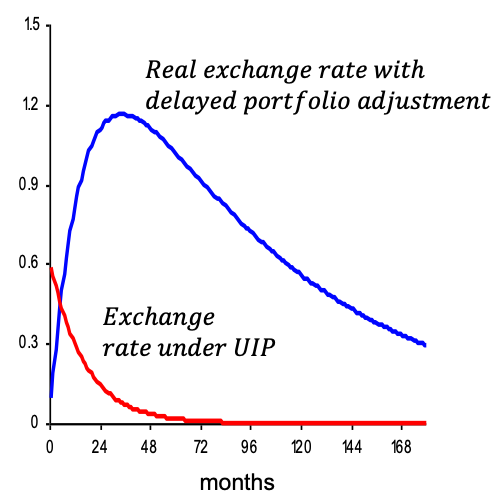
It is useful to consider a simple example to illustrate the implications of delayed portfolio adjustment for the exchange rate. Assume that the foreign interest rate suddenly increases, but the interest rate differential decreases over time. The domestic currency immediately depreciates as investors want to buy the foreign currency. If UIP holds, the domestic currency then appreciates to compensate for the higher foreign interest rate. In contrast, with delayed adjustment, the domestic currency continues to depreciate for a while, as investors gradually increase their position in foreign currency. Furthermore, the initial depreciation is smaller, since the initial portfolio change is smaller. Figure 1 shows the response of the real exchange rate (domestic currency per foreign currency) with delayed portfolio adjustment (in blue) compared to the exchange rate prevailing under UIP (in red). In this numerical example, the domestic currency depreciates over the first 35 months.
Figure 1 Impulse response to an increase in the foreign interest rate
Explaining six puzzles
Figure 1 helps to illustrate why the delayed portfolio model can solve four out of the six exchange rate puzzles we consider.
1. Delayed overshooting puzzle. The hump-shaped exchange rate response is consistent with the exchange rate response to monetary shock, which has often been reported in the literature (e.g. Eichenbaum and Evans 1995).
2. Forward discount puzzle (or Fama puzzle). The foreign currency appreciates over several months while it has a high interest rate. This means that the foreign currency has an excess return and that it can be predicted by the interest rate differential.
3. Predictability reversal puzzle. While a higher foreign interest rate typically predicts positive foreign currency returns in the short to medium run, after several quarters it tends to predict a negative return. This predictability reversal is reflected in Figure 1, as we see that the foreign currency depreciates significantly after about three years. This depreciation could more than offset the interest rate differential, and imply a negative excess return in foreign currency.
4. Engel puzzle. Engel (2016) shows that high interest rate currencies tend to be strong compared to what UIP would imply. This means that over time, the foreign currency depreciation is larger than the interest differential. In Figure 1 we see that the response of the real exchange rate with delayed portfolio adjustment is larger than the exchange rate prevailing under UIP except for the initial months and depreciates more in the long run. In our paper, we show formally the conditions under which the delayed portfolio adjustment is consistent with this puzzle.
Two other puzzles can be explained by gradual portfolio adjustment.
5. Forward guidance exchange rate puzzle. It is easy to see that UIP implies that the exchange rate is affected by undiscounted future interest rates. This means that, for example, interest rates a century from now have the same impact on the exchange rate as interest rates next month. Galí (2019) shows that this is inconsistent with the evidence – interest rates in the future have lower impact. Relating it to monetary policy, he coins this the forward guidance exchange rate puzzle. Delayed portfolio adjustment can explain this puzzle mainly because of the muted initial response of exchange rates. If the foreign interest rate is expected to increase at a future date t+k, the foreign currency should appreciate a period before, at t+k-1. But it will appreciate less than under UIP due to reduced portfolio response. The same is true at t+k-2, etc. Therefore, the exchange rate today reacts less to a future interest rate change than under UIP. In our numerical illustration, we find a monthly discount rate for future interest rates of 1.29 with gradual portfolio adjustment (instead of 1 under UIP).
6. Non-predictability of long-term bond return differential. The forward discount puzzle considers interest rates on short-term assets, typically deposits. In a recent paper, Lustig et al. (2018) examine the short-term return on long-term government bonds. They find no predictability in this case. A model with delayed portfolio adjustment with both short-term and long-term debt is consistent with this fact if we make an additional assumption of home bias for bonds. In this case, consider an increase in the foreign short-term interest rate. This implies an increase in the demand for foreign short-term assets and a foreign currency appreciation. The associated gradual portfolio adjustment away from foreign long-term bonds leads to a gradual decline in the long-term bond price and therefore its return. This return decline on the foreign long-term bond can offset the gain from currency appreciation so that there is no excess return in foreign currency longer-term assets. This only happens under gradual portfolio adjustment.
Conclusion
There is widespread evidence that many investors react slowly to new information. This has the potential to influence asset prices. In the case of exchange rates, we show that delayed portfolio adjustment is likely to be an important factor.
References
Bacchetta, P, and E van Wincoop (2010), “Infrequent Portfolio Decisions: A Solution to the Forward Discount Puzzle”, American Economic Review, 100, 837-869.
Bacchetta, P, and E van Wincoop (2019), “Puzzling Exchange Rate Dynamics and Delayed Portfolio Adjustment”, CEPR Discussion Paper no. 13839.
Eichenbaum, M S, and C L Evans (1995), “Some Empirical Evidence on the Effects of Shocks to Monetary Policy on Exchange Rates”, Quarterly Journal of Economics, 110 (4), 975-1009.
Engel, C (2016), “Exchange Rates, Interest Rates, and the Risk Premium”, American Economic Review, 106, 436-474.
Froot, K A, and R H Thaler (1990), “Anomalies: Foreign Exchange”, Journal of Economic Perspectives, 4, 179-192.
Galí, J (2019), “Uncovered Interest Parity, Forward Guidance and the Exchange Rate”, mimeo.
Lustig, H, A Stathopoulos, and A Verdelhan (2018), “The Term Structure of Currency Carry Trade Risk Premia”, working paper.