As famously argued by Pascal Lamy whilst Director General of the WTO, the world trade system has evolved from a state of protection to a state of precaution (Lamy 2012). Previously, restrictions typically took the form of traditional bilateral measures such as tariffs, with the goal of ‘protecting’ domestic producers. But more recently the bulk of restrictions have been country-specific policies such as sanitary and phytosanitary measures, technical barriers to trade, and domestic regulations (for example, in services) which have the ‘precautionary’ goal of addressing concerns over health, safety, and other social considerations, with the domestic consumer in mind. These latter country-specific policies are often more important determinants of trade flows than bilateral trade policies.
The structural gravity model has long been known as the workhorse model of applied international trade analysis. And for good reason. It performs remarkably well in predicting trade flows between country pairs and its theoretical foundations are plausible and consistent with the data (Anderson 2011). As such, the gravity model has been applied to study the impact of a plethora of bilateral policies, such as free trade agreement membership, on international trade.
But for all its merits, the gravity model has yet to disentangle the effects of country-specific policies on bilateral trade while simultaneously respecting its theoretical foundations.
More specifically, in estimating the gravity equation one must control for general equilibrium trade costs known as multilateral resistance (MR) terms (Anderson and Van Wincoop 2003). The typical way to do this is to include country-time fixed effects. But, once such fixed effects are included, the impact of any country-specific policy of interest is subsumed.
The literature has proposed several methods to estimate the impact of country-specific policies on trade, however each of these methods has been criticised:
- Some authors have omitted the country-time fixed effects that control for the structural MRs, so that they can include country-specific regressors in their own right. However, this leads to omitted variable bias, coined the ‘gold medal mistake’ by Baldwin and Taglioni (2006).
- Other authors have constructed bilateral terms from the country-specific variables of interest so that they can still control properly for the structural MR terms. But, as discussed in Heid et al. (2020), this approach can also be problematic: either the impact of the bilateral term cannot be identified due to perfect collinearity with the country-time fixed effects, or it can be identified due to functional form assumptions, but the interpretation of the resulting estimates is challenging. To get around this problem, they rely on the theory-consistent use of domestic trade flows to identify the effects of non-discriminatory trade policies.
- Most recently, Beverelli et al. (2018) use the same idea to identify country-specific policies in a structural gravity setting. However, their methods only deliver estimates of the differential impact on international relative to domestic trade and cannot identify the full effect of country-specific policies, including the uniform impact on both domestic and international trade.
In a recent paper (Freeman et al. 2021) we propose a solution to this problem and present new methods to estimate the full effect of country-specific policies on bilateral trade flows.
In addition to enabling us to estimate the full impact of any country-specific policy on bilateral trade, our methodology also brings another important benefit to the table: it allows us to estimate the trade elasticity, which is the single most important parameter for welfare analysis (cf. Arkolakis et al. 2012), at various levels of disaggregation and without the need for tariff and/or price data. A by-product of the analysis is that we obtain an estimate of the trade elasticity for services, which is typically challenging to estimate given that services trade is not taxed in the same way that goods trade is via tariffs.
Methodology in a nutshell
We capitalise and extend on the classic work of Anderson and van Wincoop (2003) and Redding and Venables (2004) to derive a two-stage estimating procedure that allows to identify the full impact of country-specific policies on bilateral trade flows and to recover the trade elasticity at any level of disaggregation.
In stage one, we (i) apply the latest developments in the structural gravity literature to obtain estimates of bilateral trade costs and trade policies in the presence of exporter-time and importer-time fixed effects; and (ii) use the first-stage gravity estimates to construct the structural MRs. Then, in stage two, we rely on theory to replace the country-time fixed effects from the first stage with country-specific variables, including the MR terms that we recover from the first stage. This allows us to estimate the impact of any country-specific variable of interest while adhering to theory. Importantly, the estimates of the coefficients on the structural MRs enable us to recover the trade elasticity parameters.
Since our theoretical assumptions are consistent with those of Arkolakis et al. (2012), our framework and empirical procedures are representative of a very wide class of trade models.
Country-specific R&D expenditure boosts bilateral trade, but effects vary by type
We bring our methodology to the data by quantifying the impact of country-specific research and development (R&D) expenditure on international trade. Specifically, we use four measures of gross R&D expenditure (total, higher education, business enterprise, and government), and decompose the impact of each R&D type into: (i) a differential effect on international relative to domestic sales; and (ii) a uniform impact on trade regardless of whether it is domestic or international.
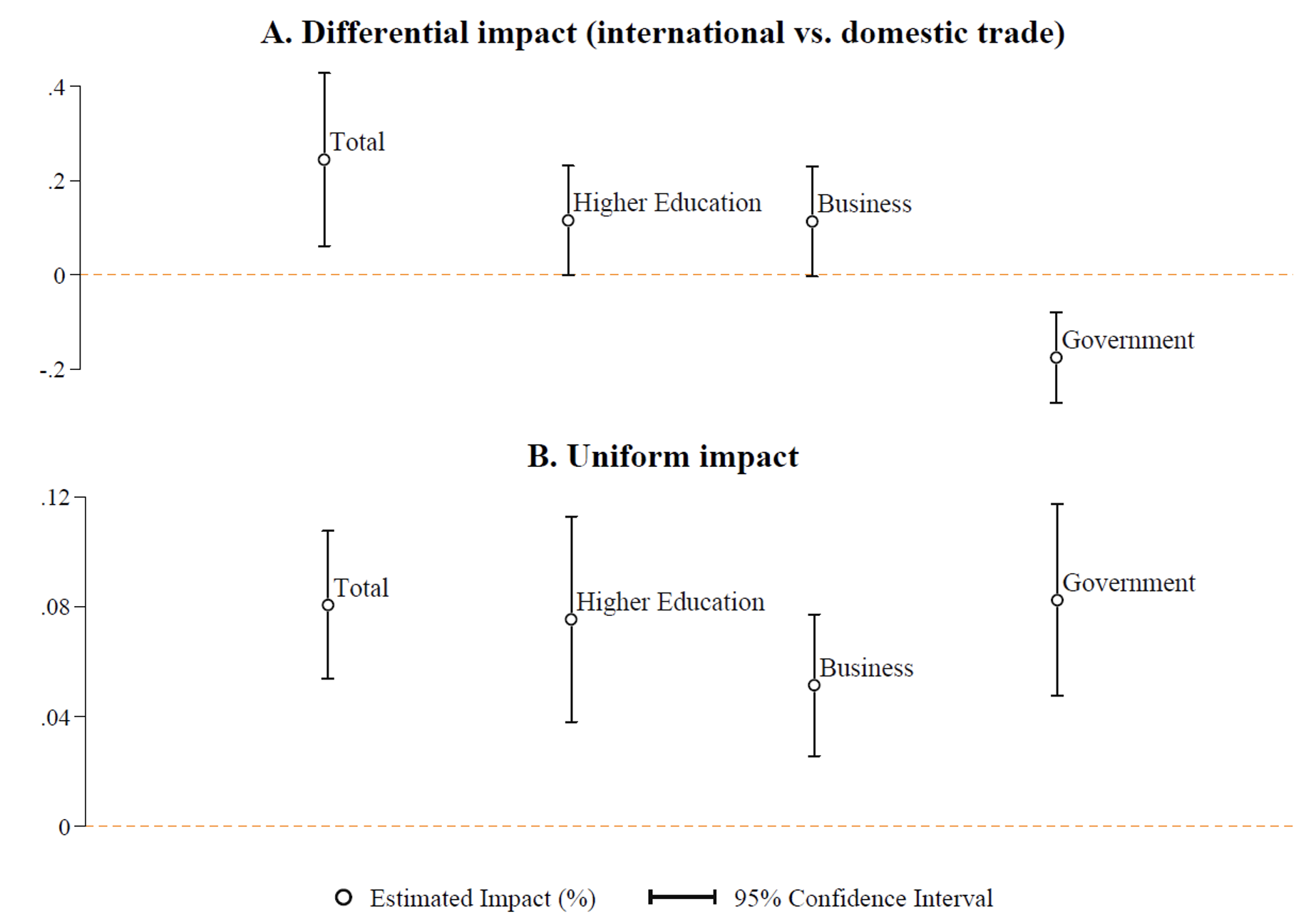
As Figure 1 shows, there is a positive and significant effect of R&D expenditure on trade, which disproportionately promotes international relative to domestic trade (Panel A). Indeed, the point estimate for “Total” implies that at 10% increase in total R&D expenditure is associated with a 2.4% increase in international (versus domestic) trade. Nonetheless, the uniform impact of R&D on sales is also positive and significant (Panel B) – an intuitive finding as we would expect that innovation in R&D should promote efficiency. In combination, summing estimates from Panels A and B imply that a 10% increase in total R&D expenditure translates into roughly a 3% increase in total bilateral trade.
Figure 1 The impact of R&D expenditure on bilateral trade (percent)
We also document significant heterogeneity by type, with positive and significant estimates of the effect of R&D expenditure in higher education and business enterprise, but a negative estimate of the impact of government allocations on international relative to domestic trade, which outweighs the uniform effect in Panel B. We find the opposing results with respect to government allocations provoking but also intuitive as one might expect some ‘home bias’ in government spending.
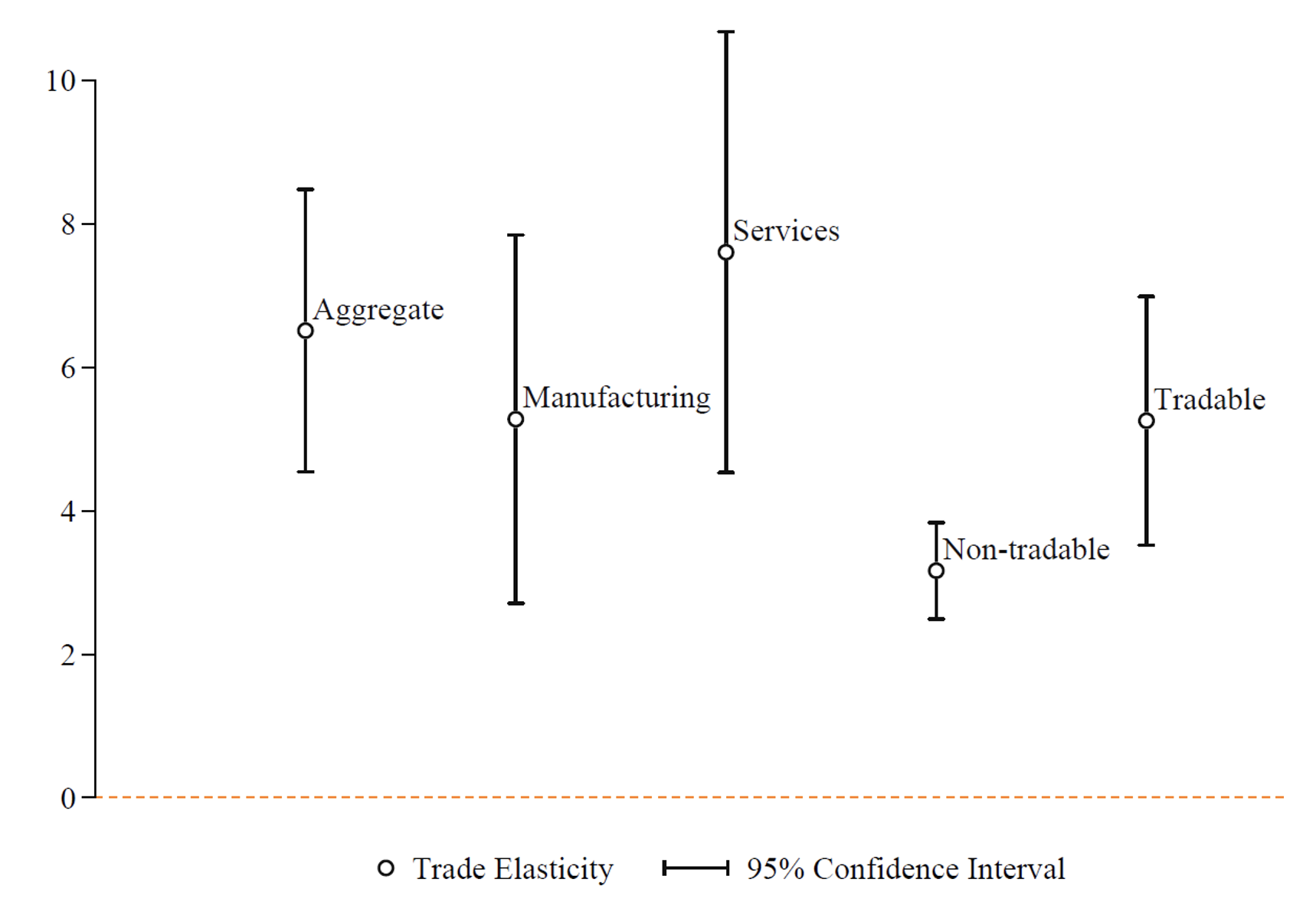
The services trade elasticity is roughly 45% than that for manufacturing
We use our methodology to recover trade elasticity parameters from the second-stage estimates of the coefficients on the structural MRs.
As shown in Figure 2, in addition to the aggregate trade elasticity we recover disaggregate elasticities for manufacturing and services, as well as tradable and non-tradable goods and services sectors. An important insight from our analysis is that we obtain novel estimates of the services trade elasticity of 7.60, which is roughly 45% larger than that for manufacturing. Given the nature of services, which are more substitutable than manufacturing goods, we find this result intuitive. Nonetheless, it sheds new light on the nature of the substitutability of services trade which is often hard to measure due to data constraints on prices and tariffs. We also find plausible and encouraging (with respect to our methods) the significantly larger trade elasticity estimates for tradable relative to non-tradable sectors.
Figure 2 Trade elasticity estimates
Summing up
We build on the natural progression of several generations of gravity literature to make two related contributions. On the one hand, we propose methods that will enable researchers and policy makers to assess the impact of any country-specific policy on bilateral trade without breaking from the underlying theory behind the structural gravity model. This is particularly poignant in today’s world where country-specific policies like technical barriers to trade, subsidies, sanitary and phytosanitary measures, and a range of non-tariff measures have outpaced bilateral policies. On the other hand, our methodology will permit researchers to easily and directly estimate trade elasticities at their preferred levels of disaggregation (including for services), for their relevant country and time samples, and without the need to rely on price and tariff data.
References
Anderson, J E (2011), “The gravity model”, Annual Review of Economics 3(1): 133-160.
Anderson, J E and E Van Wincoop (2003), “Gravity with gravitas: A solution to the border puzzle”, American Economic Review 93(1): 170-192.
Arkolakis, C, A Costinot and A Rodríguez-Clare (2012), “New trade models, same old gains?”, American Economic Review 102(1): 94-130.
Baldwin, R and D Taglioni (2006), “Gravity for dummies and dummies for gravity equations”, NBER Working Paper No. 12516.
Beverelli, C, A Keck, M Larch and Y V Yotov (2018), “Institutions, trade and development: A quantitative analysis”, CESifo Working Paper No. 6920
Freeman, R, A Theodorakopoulos, M Larch Y V Yotov (2021), “Unlocking new methods to estimate country-specific trade costs and trade elasticities”, Bank of England Staff Working Paper No. 951.
Heid, B, M Larch and Y V Yotov (2021), “Estimating the effects of non-discriminatory trade policies within structural gravity models”, Canadian Journal of Economics 54(1): 376-409.
Lamy, P (2012), Speech at the launch of the World Trade Report 2012, 16 July.
Redding, S J and A J Venables (2004), “Economic geography and international inequality”, Journal of International Economics 62(1): 53-82.