The Great Recession has produced falling real wages (Elsby et al. 2013). There has not been the ‘usual’ unemployment reaction. As such, it may seem that the wage inflation/unemployment trade-off has shifted. Our recent work suggests otherwise (Castle and Hendry 2013).
Huge changes in real wages have occurred over the period 1860–2011: real wages have risen over twelve-fold, and nominal wages almost 70,000%. Laws, technology, industrial composition, wealth distribution, housing tenure, social structure, and so on are unrecognisably different from 1860. Despite these changes, we find empirical evidence of a constant relationship between real wages and productivity, allowing for other influences, including over the recent ‘Great Recession’.
The modelling framework used in addressing such questions is fundamental. Our approach requires all relevant variables, dynamics, outliers, shifts, and non-linearities to be modelled jointly for a coherent empirical economic model (we provide an example below based on Castle and Hendry 2013). Any omitted substantive feature will result in erroneous conclusions, as other aspects of the model attempt to proxy the missing information.
At first sight, allowing for all these aspects jointly seems intractable. But help is at hand with the power of a computer. We utilise the automated model selection algorithm Autometrics (see Doornik 2009) that can handle more candidate variables than observations, including extensive dynamics, possible outliers and structural breaks, and many forms of non-linearity jointly. The algorithm has many excellent properties as described in Castle et al. (2011).
Explaining the theory
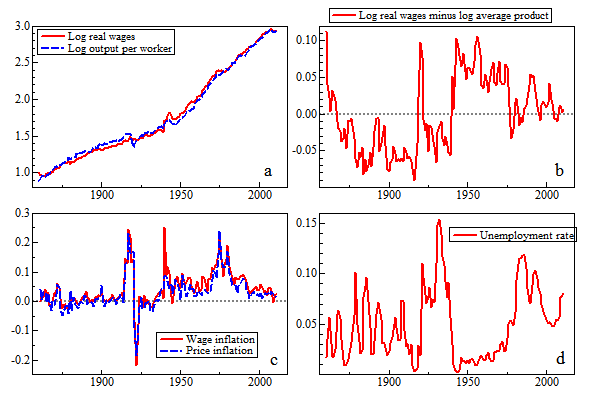
Neoclassical theory suggests that the real wage is driven by the real marginal revenue product of labour, which we proxy by output per worker given the evidence for a constant-returns technology at the aggregate level. Figure 1a records log real wages and log output per worker over the last 150 years. Although there are some substantial deviations between the two series, they move closely together. Their difference, which measures labour productivity (corrected for the sample mean), forms the equilibrium correction mechanism for the model, imposing full adjustment of real wages to productivity in the long run. This is also a measure of labour’s share in national income, and Figure 1b demonstrates that this is non-trending.
Figure 1 Real wages and output per person, wage and price inflation, and the unemployment rate
To compute real wages, price deflation is often by a consumer price index (CPI) for wages and a producer price index (PPI) for output, which adds a ‘wedge’ to wage determination models. For our long period, we use the GDP deflator for both. Figure 1c records wage and price inflation. There is some discussion as to whether the GDP deflator is an appropriate measure for wages, but movements in the CPI and GDP deflator have been roughly in line with each other over the last 20 years, with the GDP deflator having the advantage that it is less volatile as it measures the whole economy. Overall, the impact is likely to be small relative to the huge movements in the real wage over 150 years. Pessoa and Van Reenen (2012) provide further discussion.
Our empirical evidence suggests that the effects of trade unions, unemployment benefits (via replacement ratios), employment legislation, and so on, mainly impact on unemployment rather than the real wage. For example, trade unions may raise wages at a given firm, but goods market competition then forces it to reduce its employment. The unemployment rate is shown in Figure 1d. Dynamic adjustments have been explained by a range of factors, including staggered wage contracts, institutional factors such as labour laws, expectations, and indexation (as during the World War I). Labour markets are an important intermediary in the inflation process, but factor demands are derived from final demand, sometimes measured by unemployment, the output ‘gap’, or capacity utilisation, so final demand must be the direct determinant of price inflation.
Non-linearities affecting real wages
Two non-linearities are found to be important in determining real wage growth.
- First is the response of real wages to inflation.
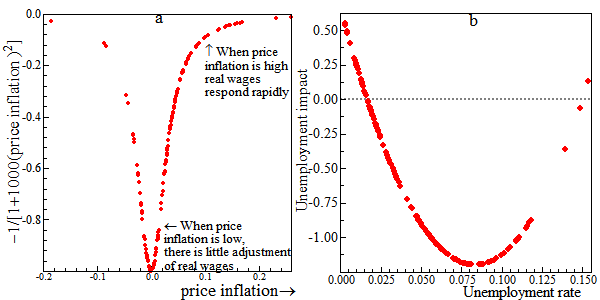
Price inflation plays two roles: directly in modelling real wages, and as a ‘catch-up’ by workers when wages have been eroded by incomplete adjustments to past inflation. The non-linear mapping that captures the evidence is U-shaped; workers become more attentive when price inflation rises, and act to prevent further erosion of their real wages, whereas employers cut nominal wages when prices fall. Such behaviour generates wage-price spirals. Figure 2a records the shape of this non-linear response, computed from the data.
Figure 2 The non-linear effects of (a) price inflation and (b) unemployment on real wages
- The second non-linear term concerns the unemployment rate.
As well as a ‘Phillips curve’ relationship, we found a substantial quadratic term. Their combined effect is shown in Figure 2b, and reveals a positive impact at low rates, an increasingly negative impact until the unemployment rate exceeds 8%, but then declines, becoming positive again around 15%.
Such outcomes probably represent interactions between changes in workers bargaining power and movements along the marginal product curve, raising real wages of those still employed as unemployment rises beyond 8%, so is only consistent with unemployment being primarily involuntary. This matches the historical data in Figure 1d, given the absence of unemployment benefits in the 19th century and near zero unemployment after the World War II. Eliasson (1999) finds a related non-linearity between unemployment and inflation in Australia.
The third aspect is jointly modelling these non-linearities with shift indicators to avoid spurious significance by their capturing outliers. Step indicators explain the higher average growth rate of real wages post World War II, namely 1.8% per annum as against 0.7% pre-1945. Although the growth in output per worker displays a similar shift, it is insufficient to explain the increase in real-wage growth, suggesting that a structural shift occurred in the workforce, partly precipitated by an increase in women in the labour market. Nielsen (2009) finds that interactions of variables with step shifts matter, whereby unemployment enters in a different functional form in different regimes. The non-linear relation here captures this varying effect of unemployment on real-wage growth. Impulse indicators for the war years allow us to model the long time series as a whole, rather than truncating the data and only considering the more recent past.
The role of productivity in explaining real wages
The short-run impact of changes in productivity on real wages is approximately 0.6, and is fairly constant over the 150 years. This implies that changes in labour productivity should feed through to real-wage growth rapidly. There is a strong equilibrium correction effect of -0.18, so past disequilibria between real wages and output per worker are adjusted back to equilibrium at about 18% per annum.
Policy analysis
Policy requires changing one variable, say x, to alter another, say y. In the simplest case, a shock to x changes y linearly. This requires both a constant causal link, and if the linear relationship has been estimated by conditioning on x, super exogeneity. These hypotheses can be tested by selecting step shift indicators in a vector autoregression for all variables that enter contemporaneously, and adding those indicators to the equation being modelled. If the added indicators are significant, then shifts in policy variables change the relationship, so the response will not be as expected. Doing so for inflation, output per person and unemployment produced 20 indicators in their equations selecting at 0.5% (so only large shifts are found), which were insignificant when added to the real-wage model, sustaining policy.
Can we explain recent real wage behaviour?
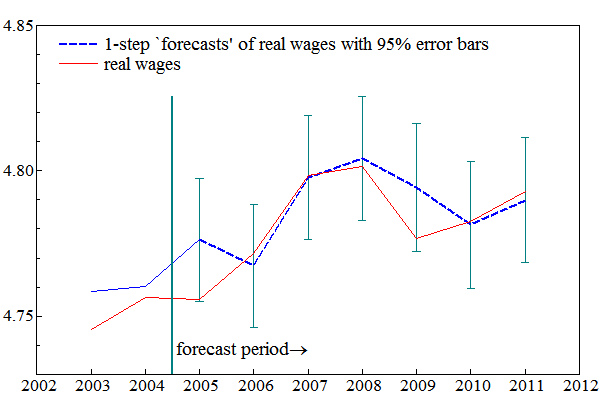
The model we propose in Castle and Hendry (2013) is a complicated non-linear specification. The forecasting literature often finds that the forecast performance of non-linear models is poor in comparison to linear models, and even more so when facing structural breaks. Using our non-linear model to make 1-step ahead conditional ‘forecasts’ over the period 2005–2011, including the Great Recession (shown in Figure 3), the model outperforms linear and naïve models, and produces an out-of-sample root mean square error of 1.05%, close to the in-sample fit of 1.04%, despite going through the deepest and longest recession since the war.
Figure 3 1-step ahead conditional ‘forecasts’ of real wages
Conclusions
The Great Recession has led to reductions in real wages, rather than the ‘usual’ unemployment reaction, partly by a reduction in hours through more shift/part-time work. As such, it may be thought that the wage inflation/unemployment trade-off has shifted. However, our model provides a constant-parameter relationship over the 150 years of data since 1860. Deflating nominal wages by the Retail Price Index (RPI) over the recession suggests a substantive fall in real wages partly because the arithmetic average computation of the RPI overstates inflation, partly because firms determine (product) real wages, so all additional price `wedges’ must be borne by wage recipients.
There are three important economic implications. First, a wage-price spiral can result from increasing reactions of wages to price inflation as inflation rises. That adds persistence to the wage–price process, creating an impression of ‘sticky inflation’. Second, real wages are primarily determined by forces different from nominal prices, consistent with the ‘Classical dichotomy’. Third, there is an additional non-linearity in unemployment, showing a falling impact on real wages as unemployment rises beyond 8%. That finding is consistent with substantial involuntary unemployment, as no evidence of any reverse relation of high real wages causing unemployment was found in the corresponding inflation model (Hendry 2001).
The analysis emphasises the need for jointly modelling dynamics, location shifts, relevant variables and non-linearities. Failing to include any of these features led to substantive mis-specifications, with included variables being insignificant in restricted formulations, yet important in more general models. Automatic model selection like Autometrics seems a viable approach to tackling all the complications jointly, even when there are more candidate variables than observations.
References
Castle, J L and D F Hendry (2013), “Semi-automatic non-linear model selection”, in N Haldrup, M Meitz and P Saikkonen (eds), Essays in Nonlinear Time Series Econometrics, Oxford: Oxford University Press.
Castle, J L, J A Doornik and D F Hendry (2011), “Evaluating automatic model selection”, Journal of Time Series Econometrics 3 (1), DOI: 10.2202/1941–1928.1097.
Doornik, J A (2009), “Autometrics”, in J L Castle and N Shephard (eds), The Methodology and Practice of Econometrics, Oxford: Oxford University Press, pp. 88–121.
Eliasson, A-C (1999), Smooth Transitions in Macroeconomic Relationships, Stockholm: Economic Research Institute, Stockholm School of Economics.
Elsby, M W L, D Shin and G Solon (2013), “Wage Adjustment in the Great Recession”, NBER WP 19478.
Hendry, D F (2001), “Modelling UK inflation, 1875–1991”, Journal of Applied Econometrics 16, pp. 255– 275.
Nielsen, H B (2009), “Comment on ‘the long-run determinants of UK wages, 1860–2004”, Journal of Macroeconomics 31, pp. 29–34.
Pessoa, J P and J Van Reenen (2012), “Decoupling of wage growth and productivity growth? Myth and reality”, Discussion paper, Resolution Foundation.
Phillips, A W H (1958), “The relation between unemployment and the rate of change of money wage rates in the United Kingdom, 1861–1957”, Economica 25, pp. 283–299.