In the wake of the Global Crisis, policymakers ran into what, until then, had seemed a merely academic possibility – the effective lower bound (ELB) on interest rates. In response, major central banks resorted to unconventional monetary policies, ranging from security market interventions to forward guidance and specific measures to support distressed banks. Ten years after the start of the Global Crisis, it is worth taking stock of central banks’ accumulated experience with these innovations, and to draw out the lessons it may provide for the design and conduct of policy going forward.
Against this background, it is key to understand whether and how unconventional measures have impacted market sentiment. We focus on the extent to which unconventional ECB policy measures have reduced the perception of crash risk in the Eurodollar exchange rate. We define tail risk as the risk that the euro weakens significantly against the dollar. We choose the exchange rate for two reasons. First, euro area breakup risk was a real market fear during the European sovereign debt crisis. As such, crash risk perceptions in the exchange rate are a good instrument to capture markets’ judgement of a further escalation of the crisis (Farhi et al. 2009). Second, the exchange rate is an important transmission channel of monetary policy (Arteta et al. 2018).
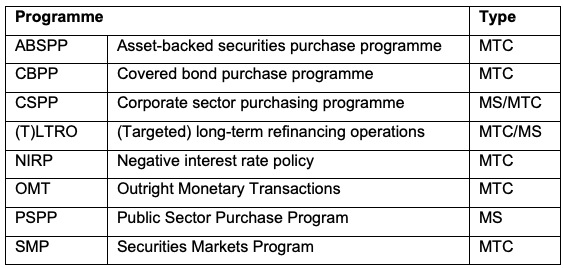
To answer this question, we use the information embedded in option prices to derive a measure of tail risk perceptions. Currency options are the most liquid options and are therefore likely to capture changes in risk perceptions timely and adequately. The analysis is based on daily data from January 2009 to December 2018 and differentiates between two types of unconventional monetary policy: measures that target the ECB’s monetary stance, and measures that target the monetary transmission channel given the monetary stance (Table 1).
Table 1 Characterisation of ECB’s unconventional monetary policy measures
Note: MS refers to monetary stance; MTC refers to monetary transmission.
Option prices contain information on the perception of tail risk by investors
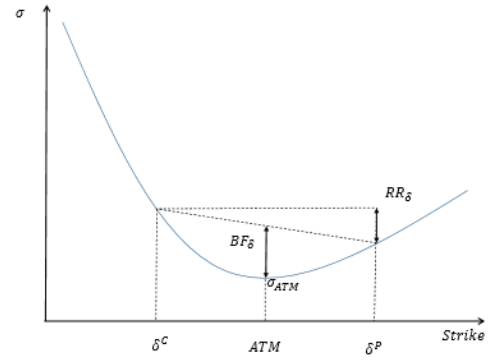
We proceed in three steps to extract information about crash risk from (out-of-the-money) Eurodollar option prices. First, we look at so-called risk reversals. Risk reversals are a measure of the cost of hedging against downside risk by capturing the extent to which put options (which offer downside protection) are more expensive than call options (which offer upside protection). Risk reversals can therefore serve as a proxy for capturing the extent to which the return distribution is asymmetric (i.e. skewed, see Figure 1).
Figure 1 Risk reversals σ (y-axis) reflect the implied volatility, δ is the option delta (x-axis)
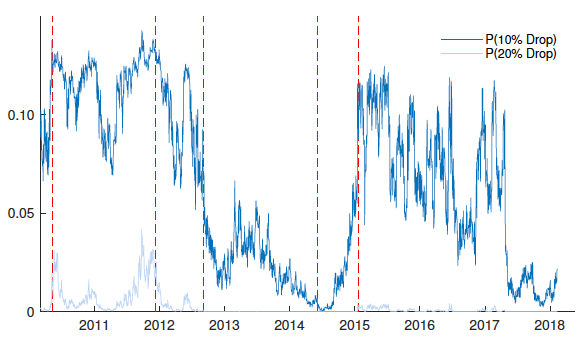
We then examine the entire distribution of future exchange rates (probability density function) using the Black-Scholes method to investigate all four moments of the distribution (mean, variance, kurtosis, and skewness). This allows for more descriptive measures of the distribution. This is important when, for instance, the variance or the kurtosis of the distribution is volatile while the skewness of the distribution remains the same. When the former is the case, the results based on the probability density functions may differ from the risk reversals. From the estimated distribution, we can calculate the probability of a specific drop – say, 10% or 20% – in the exchange rate in the coming month (Figure 2).
Figure 2 Risk-neutral probability density-based time series of a 10% and 20% crash
Note: Figure 2 shows the crash probabilities defined as a drop of 10% and 20% derived from the RNPD based on 1-month options. The y-axis is in percentage points, x-axis in years. Red dotted lines represent (from left to right) the first announcement of the SMP, the first announcement of the TLTRO programme, technical features of the OMT, the announcement to lower the DFR below 0 and the announcement of the PSPP.
As several assumptions of the Black-Scholes method (Black and Scholes 1973) are violated in reality, we also apply a method that allows for instantaneous jump risk to capture the amount of perceived crash risk in currency markets. More specifically, the Black-Scholes method revolves around the assumption of constant volatility. In order to work around this assumption, we use an explicit mixed jump diffusion risk model as outlined in Kou (2002). A benefit of the Kou model is that it does not use strict assumptions for modelling the tails of the distribution (i.e. so-called endpoint clamping), the most important part for the determination of crash risk perceptions. More specifically, this mixed jump diffusion model allows for fat-tailed distributions.
Monetary stance policies seem to be more effective in downplaying crash risk than policies aimed at restoring the transmission channel
An overarching conclusion is that policies aimed at changing the relative supply within different asset classes have an impact on perceived crash risk, while measures aimed at easing financing costs do not. Our paper thereby emphasises the importance of tail risk indicators, since kurtosis and skewness indicators sometimes tell a different story than the central tendency (i.e. the mean) of a specific distribution. More specifically, in this column we shed light on two of the main findings that emerge from our analysis (Olijslagers et al. 2019). First, we find that the Securities Markets Program (SMP) – which focused on supporting monetary transmission by intervening in particular markets for sovereign debt – actually led to higher tail risk perceptions in the Eurodollar market. Second, we document that announcing policies in general terms, without precisely describing what exactly they entail, did not instantly move asset markets. In some cases, this even moved them in the wrong direction.
All crash risk measures point to an increase in tail risk perceptions surrounding the SMP programme
Not all unconventional monetary policies conducted by the ECB have been successful in terms of diminishing crash risk perceptions in the euro area. We find that the SMP programme actually led to higher downside risk perceptions. In particular, the programme did not really lower tail risk perceptions, and according to the jump-diffusion risk model it actually led to significantly higher risk perceptions. A possible explanation is that the SMP lacks conditionality and can in the longer term lead to more fragmentation (which contributes to the perception of crash risk). Reis (2017) provides another explanation for this result by indicating that mismatches between the composition of asset purchases and the capital key of the ECB governing its distribution of dividends signal ultimately unsustainable redistribution. According to this theory, the asset purchases might, in the short term, calm down markets (and lower tail risk perceptions), but in the long term – due to their unsustainable nature – lead to higher tail risk perceptions as they possibly increase crash risk (by an increase in redenomination risk).
The OMT programme led to lower tail risk perceptions only when the details were made clear enough
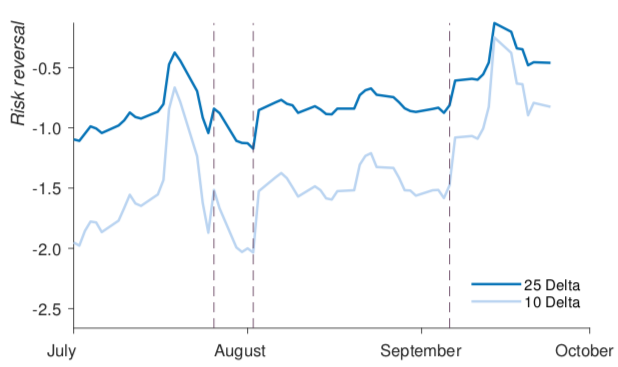
In the midst of the European sovereign debt crisis, Draghi tried to calm market fears by assuring that the ECB would do “whatever it takes" to preserve the euro. Although this speech is often considered to have downplayed market fears, Figure 3 shows that Draghi’s speech initially did not deliver upon market expectations (in fact it shows a jump in tail-risk perceptions in the days after Draghi’s statement). More specifically, tail risk in the Eurodollar increased substantially afterwards (i.e. a lower risk reversal implies a higher tail risk). Seemingly, investors thought the statement contained too little information to scale down their hedging of downside risks in the Eurodollar exchange rate. The finding is even more remarkable as the price of the euro against the dollar jumped by 1.1% after the speech. So, the value of the euro (which reflects the mean price) did increase, but this increase did not result in lower crash risk perceptions.
Figure 3 Risk reversals surrounding the OMT programme announcements
Note: Figure 3 displays the 10 and 25 delta risk reversal (RR = IVcall – IVput for same delta) based on 1-month options from July-October 2012. The dotted lines represent the announcement days (from left to right) of the “Whatever it takes” speech, further hints on OMT, and the launch of the OMT.
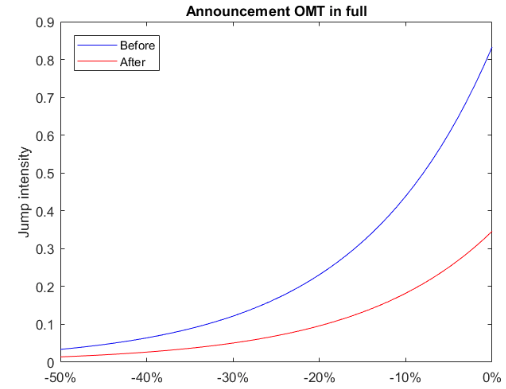
The market view changed when Draghi announced that the ECB was exploring options to support sovereign debt markets. This occurred when the final framework of the OMT programme was published in September 2012. In the days following this announcement, the risk reversal moved close to zero, signalling a nearly identical probability of the euro moving up or down. These findings are underlined by our jump-diffusion risk model (Figure 4) and also occurred – although less extreme – after the announcement of the Public Sector Purchase Program. At the same time, these observations substantiate our main conclusion that unconventional monetary policy only directly moves security markets when the relevant details are announced.
Figure 4 Jump risk model points to diminished crash risk perceptions after the full OMT announcement
Note: Figure 4 shows the estimated jump intensities. The blue line represents the intensity before the announcement and the red line the intensity after the announcement of the OMT in full detail (6-9-2012). On the x-axis the potential size of a crash is given. The (risk-neutral) probability of a drop larger than x% within one year is the area below the intensity curve to the left of point x%.
Authors’ note: The views expressed are those of the authors and do not necessarily reflect those of the Eurosystem or De Nederlandsche Bank.
References
Arteta, C, M A Kose, M Stocker, and T Taskin (2018), “Implications of negative interest rate policies: An early assessment”, Pacific Economic Review 23(1): 8-26.
Black, F, and M Scholes (1973), “The pricing of options and corporate liabilities”, Journal of Political Economy 81(3): 637-654.
Farhi, E, S P Fraiberger, X Gabaix, R Ranciere, and A Verdelhan (2009), “Crash risk in currency markets”, NBER Working Paper no. 15062.
Hattori, M, A Schrimpf, and V Sushko (2016), “The effects of quantitative easing on interest rates: Channels and implications for policy”, American Economic Journal: Macroeconomics 8(2): 111-136.
Kou, S G (2002), “A jump-diffusion model for option pricing”, Management Science 48(8): 1086-1101.
Olijslagers, S W J, A Petersen, N J de Vette, and S J G van Wijnbergen (2019), “What Option Prices tell us about the ECB’s Unconventional Monetary Policies”.
Reis, R (2017), “Can the central bank alleviate fiscal burdens?”, CEPR Discussion Paper no. 11736.