The most successful and most widely used empirical model of international trade, the gravity equation, has long been criticised for not being able to capture the decreasing impact of distance on international trade flows over time. This failure is known as the ‘distance puzzle in trade’ (Disdier and Head 2008). More broadly, Coe et al. (2002) define “the failure of declining trade-related costs to be reflected in estimates of the standard gravity model of bilateral trade” (p.1) as the ‘missing globalisation puzzle’. Yet another prominent puzzle, spanning across the international trade and macroeconomics literatures, is known as the ‘trade versus macro elasticity puzzle’ or the ‘international elasticity puzzle’ (Ruhl 2008). It pertains to the gap between the estimates of the trade elasticity from the two literatures.
In a new paper (Anderson and Yotov 2020), we propose a short-run gravity model that simultaneously resolves all three of the above-mentioned puzzles. In broader perspective, the short-run gravity model opens a way to introduce short-run adjustment and considerations to current popular practice in general equilibrium trade policy simulation analysis. This is demonstrated with the simulation of a counter-factual long-run equilibrium allocation of marketing capital/bilateral capacity in 2006. World real manufacturing income gains are under 1% in 2006. The same marketing capital allocations in earlier years imply larger gains, up to more than 2% in 1988.
Short-run gravity
Our structural short-run gravity model nests its standard (long-run) gravity counterpart, which is representative of a wide array of theoretical micro-foundations (Arkolakis et al. 2012). Two novel theoretical components – (1) a bilateral capacity/marketing capital term and (2) a structural elasticity parameter – distinguish the short-run gravity model from its long-run counterpart. These exact components are also responsible for solving the empirical puzzles. We discuss each of them next.
Solving the distance and missing globalisation puzzles
The bilateral capacity variable, ‘marketing capital’, is interpreted as origin-destination-specific investment in bilateral trade links relative to aggregate origin-specific investment in trade links,1 and it resolves the invariance (distance/missing globalisation) puzzles. Short-run gravity intuitively implies that trade costs rise with volume due to diminishing returns to a variable factor paired with the bilaterally specific capacity/marketing capital. Changes in bilateral capacity over time are omitted in standard gravity regressions. The short-run gravity model suggests that missing globalisation is the missed reallocation of marketing capital toward cross-border trade.
To identify the reallocation of marketing capital toward cross-border trade, we employ two alternative approaches.2 First, a traditional gravity model with standard proxies (e.g. distance, contiguity, common official language, etc.) for bilateral trade costs is augmented with time-varying distance effects on international trade relative to domestic trade to reflect reallocation of marketing capital toward cross-border trade. This approach targets the ‘distance puzzle’ and the ‘missing globalisation puzzle’. The model is fitted to manufacturing data for 52 countries over the era of globalisation, 1988-2006.
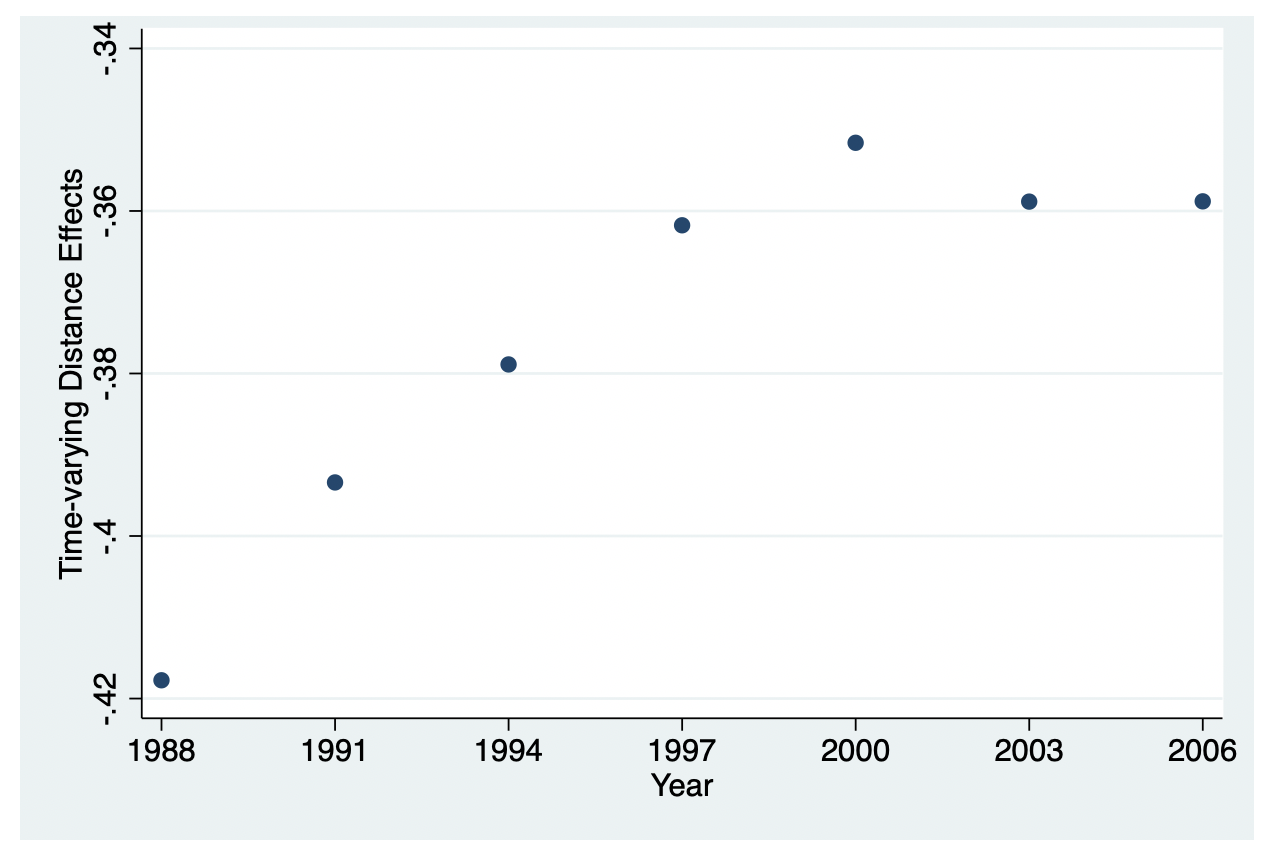
Figure 1 plots the estimates of the time-varying effects of distance on trade. The figure depicts a trend of falling absolute value of distance elasticities of bilateral trade.
Figure 1 A solution to the distance puzzle of trade
The steep and almost linear initial slope reflects the fast and steady globalisation pace during the 1990s. The spike in 2000-2001 can be interpreted as a reflection of the global recession during this period or of the accession of China in the World Trade Organization (WTO). Finally, the subsequent flat shape in the early 2000s probably captures the weakening globalisation forces during the move of the world economy toward the deep global recession of 2008. Based on the estimates in Figure 1, the overall change in the impact of distance on international trade between 1988 and 2006 is a statistically significant decrease of 14.1% (standard error 1.908), offering strong support for the proposed theoretical solution of the ‘distance puzzle’.
We employ a second, more comprehensive, approach to capture the reallocation of marketing capital toward cross-border trade. Specifically, we implement a dummy variable estimation, where all time-invariant bilateral trade costs are captured through country-pair fixed effects and the relocation of marketing capital toward cross-border trade is captured through the estimates of time-varying international border dummy variables. The more comprehensive nature of modelling trade costs in this specification targets the ‘missing globalisation puzzle’.
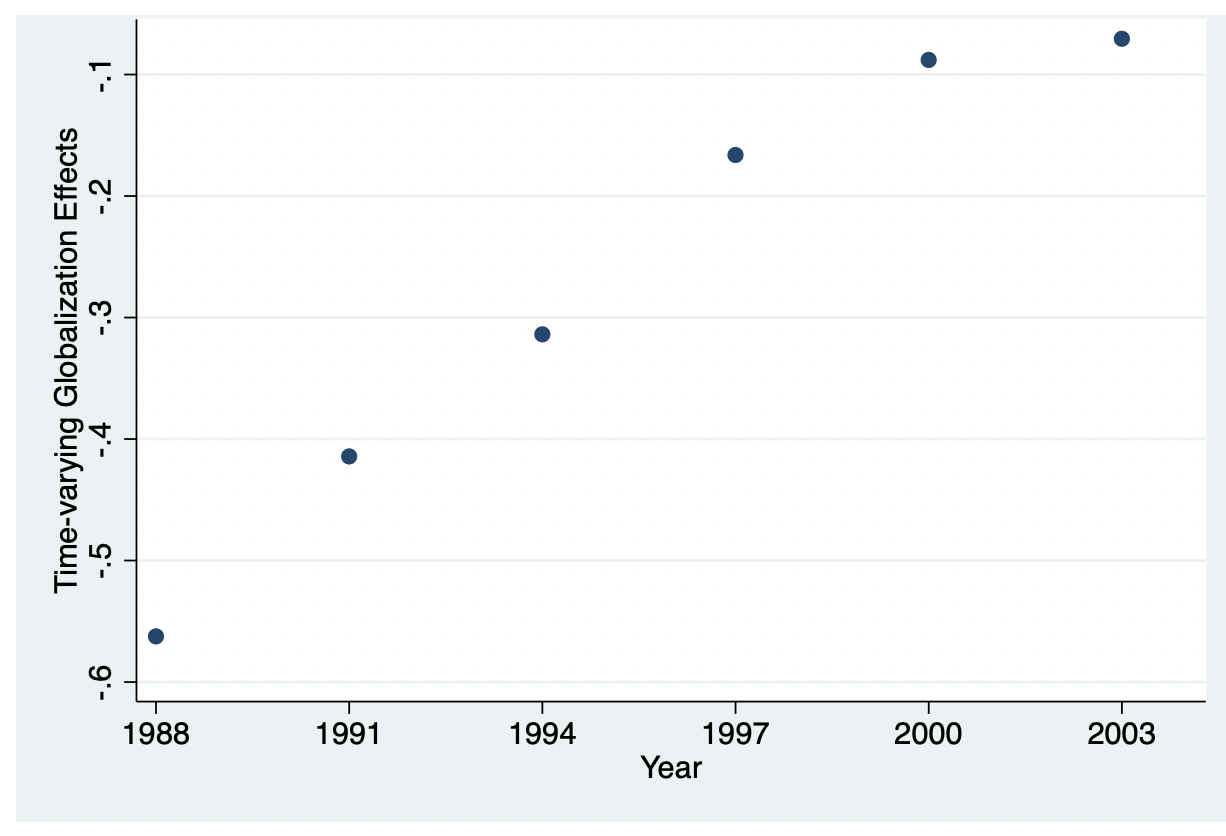
Figure 2 plots the estimates of the time-varying international border dummies. Due to the use of country-pair fixed effects (which absorb all time-invariant bilateral trade costs), the estimates of the time-varying border effects are relative to a year of choice, 2006. Once again, the figure depicts a clear globalisation trend captured by the falling estimates of the international border effects. As with the time-varying distance estimates from Figure 1, the steeper initial slope reflects the fast globalisation pace of the 1990s, while the flattening shape in the second half of the figure reflects slowing globalisation toward the global recession of 2008. The 1988 border estimate of -0.562 (standard error 0.031) implies that globalisation, interpreted as growing cross-border trade capacity, increased the world trade volume by a remarkable 75.5% (standard error 5.448) between 1998 and 2006.
Figure 2 A solution to the missing globalisation puzzle
In Anderson and Yotov (2020), we demonstrate that both (non-declining distance elasticity and missing globalisation) puzzles remain when the model is estimated in their sample without proper account for investment in cross-border capacities. Effectively, we show that globalisation was hiding in the origin-time fixed effects of standard gravity estimations. Explicitly controlling for the theory-motivated efficient reallocation of marketing capital resolved both time-invariance puzzles.
Solving the international elasticity puzzle
The second theoretical component that distinguishes our short run gravity model of from standard long-run gravity model is a micro-founded incidence elasticity parameter, which is interpreted as the buyers' short-run incidence elasticity, i.e. the fraction of a 1% rise in trade cost that is paid by buyers in short-run equilibrium.3 With the help of this parameter, the short-run gravity model generates a simple prediction about the relationship between short-run (SR) and the long-run (LR) elasticities for any gravity variable (e.g. bilateral distance, free trade agreements, etc.), including the trade elasticity, which is the coefficient on tariffs in the structural gravity model.
Using the manufacturing database, two alternative estimation methods (a reduced-form dynamic approach and an ad-hoc structural dynamic model) separately and simultaneously deliver estimates of the incidence elasticity parameter, which are close to each other. Robustness checks with an alternative database yield similar results.
Our preferred estimate of the incidence elasticity parameter is 0.27, and it implies that the short-run trade elasticity should be one-quarter of its long-run counterpart. The long-run trade elasticity estimates from the trade literature usually range between 4 and 12 (Head and Mayer 2014), while the corresponding short-run elasticities from the international real business cycle (IRBC) macro literature are usually between 1 and 2 (Heathcote and Perri 2002). Thus, the short-run gravity model reconciles the difference between the trade and the macro trade elasticities through the structural parameter, and explains why the long-run elasticities from the trade literature are much larger than short-run estimates used in the macro literature and brings them close to each other. Thus, solving the ‘international elasticity puzzle’.
How far might globalisation go?
In addition to resolving the above-mentioned empirical puzzles, the short-run gravity model raises a new question - how far might globalisation (defined as marketing capital reallocation) go? To answer, we employ the fully-fledged general equilibrium structure of short-run gravity along with the estimate of the key incidence parameter and other gravity parameters. With these tools, we quantify the potential real income gain from the long-run allocation of marketing capital - the efficient allocation absent adjustment costs. The short-run static equilibrium is compared to the long-run counterfactual static equilibrium in 2006, the last year in the sample, holding constant the 2006 endowments, preferences, trade costs, and parameters and reallocating marketing capital in each origin such that returns are equalised across destinations.
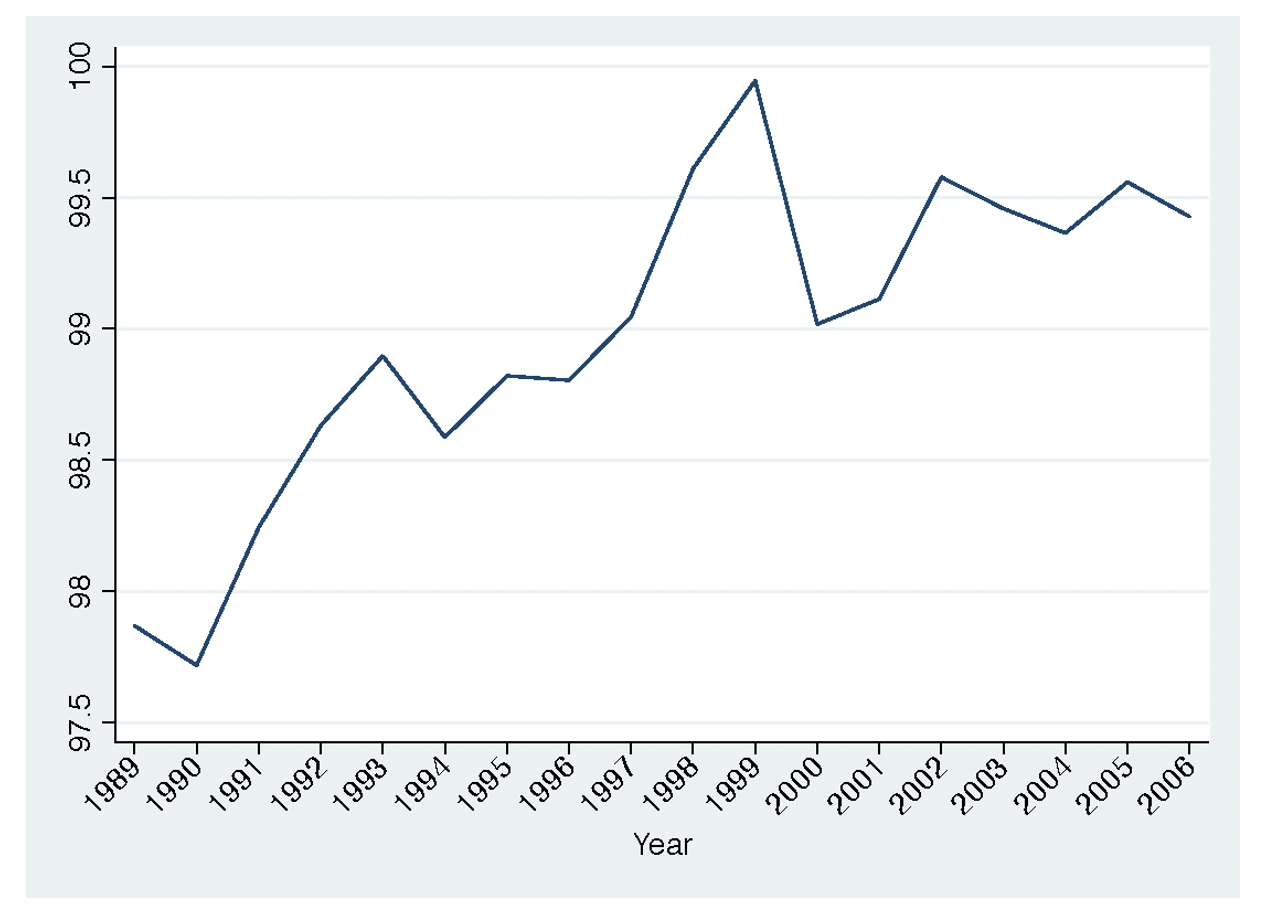
Figure 3 presents the main findings. Gains from long run globalisation are significant but become relatively small toward the end of the globalisation era: world real manufacturing income gains range from 2.15% in 1989 to 0.57% in 2006. The trend suggests movement toward long-run allocation. Also consistent with the reallocation story, the shortfall of cross-border marketing capital rises following major liberalisation events in the mid-1990s (the WTO, NAFTA and the eastward expansion of the EU) and 2000 (the permanent WTO accession of China). Through the lens of the model, the ‘permanent’ implementation increases the gap between current cross-border marketing capital allocation and its counterfactual long-run allocation in 2006.
Figure 3 Convergence from the short run to the long run
The short-run gravity model suggests several extensions. The model can be applied at the firm level, where indeed its assumption of a common Cobb-Douglas distribution ‘production’ function is most natural. Another potential extension is the explanation of income inequality. The rents to destination-specific capacity may be linked with factor market frictions and costs of adjustment that induce income inequality within firms and across firms in a sector. The model can also be nested within a multi-sector general equilibrium setting, where the stock of labour and human capital are simply the sectoral allocations, possibly subject to adjustment frictions. Empirical implementation and tests of the short run and long run extensive margin implications of short-run gravity is an important challenge. The results suggest the usefulness of short-run gravity in modelling bilateral trade even in eras where dynamic adjustment may be more complex. Finally, from a broader perspective, the short-run gravity model opens a way to introduce short-run adjustment considerations to current practice in general equilibrium trade policy simulation analysis.
References
Anderson, J E and Y V Yotov (2020), “Short Run Gravity”, Journal of International Economics 126.
Arkolakis, C, A Costinot and A Rodríguez-Clare (2012), “New Trade Models, Same Old Gains?”, American Economic Review 102(1): 94-130.
Bergstrand, J H, M Larch and Y V Yotov (2015), “Economic Integration Agreements, Border Effects, and Distance Elasticities in the Gravity Equation”, European Economic Review 78: 307-327.
Chaney, T (2014), “The Network Structure of International Trade", American Economic Review 104(11): 3600-34.
Coe, D, A Subramanian, N Tamirisa and R Bhavnani (2002), “The Missing Globalization Puzzle”, IMF Working Paper No. 171.
Disdier, A-C and K Head (2008), “The Puzzling Persistence of the Distance Effect on Bilateral Trade”, Review of Economics and Statistics 90(1): 37-48.
Head, K, T Mayer and J Ries (2010), “The Erosion of Colonial Trade Linkages after Independence”, Journal of International Economics 81(1): 1-14.
Head, K and T Mayer (2014), “Gravity equations: workhorse, toolkit, and cookbook”, in G Gopinath, E Helpman and K S Rogoff (eds.), Handbook of International Economics Volume 4, Chapter 3, Oxford: Elsevier Ltd.
Heathcote, J H and F Perri (2002), “Financial Autarky and International Business Cycles", Journal of Monetary Economics 49(3): 601-627.
Mion, G and L D Opromolla (2014), “Managers' mobility, trade performance, and wages”, Journal of International Economics 94(1): 85-101.
Ruhl, K J (2008), “The International Elasticity Puzzle", unpublished manuscript.
Yotov, Y V (2012), “A Simple Solution to the Distance Puzzle in International Trade", Economics Letters 117(3): 794-798.
Yotov, Y V, R Piermartini, J-A Monteiro and M Larch (2016), “An Advanced Guide to Trade Policy Analysis: The Structural Gravity Model”, Geneva: UNCTAD and WTO.
Endnotes
1 Bilateral capacity here is a notion consistent with the network link dynamics modeled by Chaney (2014), with the link between managers' experience in previous firms and the export performance of their current company described by Mion and Opromolla (2014), as well as with the ‘marketing capital’ notion of Head et al. (2010).
2 In each case, the gravity model is estimated following the latest best estimation practices from the related literature. See Head and Mayer (2014) and Yotov et al. (2016). The reduced-form econometric specifications with intra-national trade flows are resolve the invariance puzzles are similar to those from Yotov (2012) and Bergstrand et al. (2015). The important difference is that we offer theoretical solutions to the ‘distance’ and ‘missing globalisation’ puzzles.
3 The incidence elasticity parameter measures the proportion by which the short run trade elasticity is reduced from the long run trade elasticity. The incidence parameter derives from the equilibrium of short run supply and demand. The supply side is parameterized with a short run supply elasticity based on a Cobb-Douglas technology of production-cum-distribution. The demand side is parameterized with the CES demand system.