We are all macroprudentialists now, as Claudio Borio wrote on Vox a decade ago (Borio 2009). One pillar of macroprudential regulation is additional capital requirements for global systemically important banks (G-SIBs). The failure of such banks would generate large negative externalities, so it makes sense to reduce the likelihood of their failure by setting higher capital requirements. Our research points to efficiency gains from applying a similar logic to liquidity requirements (Aldasoro and Faia 2016, Ferrara et al. 2019).
A key tool of liquidity regulation is the liquidity coverage ratio (LCR). Defined by the Basel Committee on Banking Supervision (BCBS), the objective of the LCR is to reduce individual banks’ exposure to liquidity risk by requiring them to hold enough liquid assets to cover outflows (net of inflows) over one month of stressed conditions (BCBS 2013).1 The LCR thus prevents banks from underinsuring against liquidity risk by offsetting the moral hazard from deposit insurance and central banks’ lender of last resort facilities (Farhi and Tirole 2012, Stein 2013).
However, as a microprudential standard, the LCR applies uniformly across banks; it does not take externalities into account. Where detailed data allow regulators to measure externalities, macroprudential authorities can differentiate liquidity requirements to reflect the outsized contribution of systemically important banks to liquidity contagion. In distinct settings, Aldasoro and Faia (2016) and Ferrara et al. (2019) reach a common conclusion: setting minimum liquidity requirements in proportion to banks’ systemic importance could improve the efficiency of regulation in ensuring financial stability.
Insights from a formal model (part I)
Aldasoro and Faia (2016) develop a network model featuring contagion on both the asset and liability sides of banks’ balance sheets. In this model, banks optimise their balance sheet structures subject to regulatory constraints. Interactions between banks take place directly, through interbank interconnections, and indirectly, via common exposures to external illiquid assets (i.e. assets other than cash and reserves and interbank assets). On top of these two interactions, the model captures liquidity hoarding and endogenous bank runs triggered by information coordination (akin to the global games literature). When external assets are hit by shocks, all contagion channels interact with each other to generate systemic risk. The model is calibrated to the European interbank network by a simulated method of moments approach.
In this setting, three policy regimes are compared: no liquidity requirement; a microprudential requirement (uniform across banks); and a microprudential requirement with a macroprudential overlay (with systemically important banks subject to higher expected outflows than less important banks).
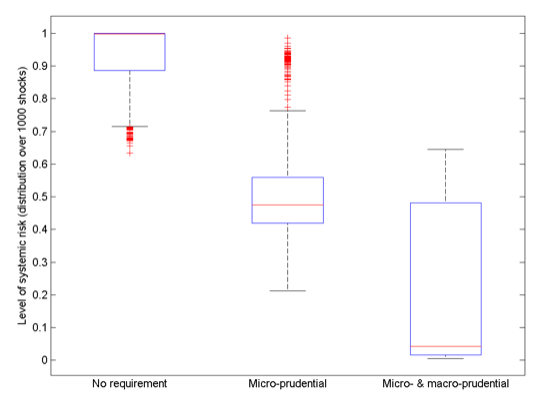
In Figure 1, the three box-and-whiskers plots show the distribution of systemic risk over 1,000 shock realisations under each policy regime. The microprudential requirement substantially reduces systemic risk (measured as the share of assets lost following a shock) relative to no requirement. Systemic risk drops further, and by a similar amount, when the microprudential requirement is given a macroprudential twist. This result is achieved simply by skewing the distribution of liquidity requirements towards systemically important banks – with no change in aggregate liquidity requirements. This quantifies the efficiency gain from a macroprudential approach to the cross-section of liquidity requirements.
Figure 1 Systemic risk under three regimes for liquidity requirements
Source: Aldasoro and Faia (2016).
Note: Each box summarises the distribution of systemic risk over 1,000 shock realisations. Red lines indicate the median of the distribution; blue boxes range from the lower quantile (25%) to the upper quantile (75%); whiskers range from minimum to maximum values (excluding outliers, which are captured by the red crosses).
Insights from a formal model (part II)
Ferrara et al. (2019) focus on the propagation of illiquidity in the interbank funding market. In this market, one bank’s outflow of cash is another’s inflow: failure to roll over short-term funding or repay obligations when they fall due creates an externality by hurting counterparties’ cash inflows. ‘Systemic illiquidity’ occurs when this externality generates a network effect through cascading funding shortfalls. Systemic illiquidity is modelled by extending the dynamic programming algorithm of Eisenberg and Noe (2001) to multiple time periods. The model is calibrated to Bank of England data on the UK interbank network.
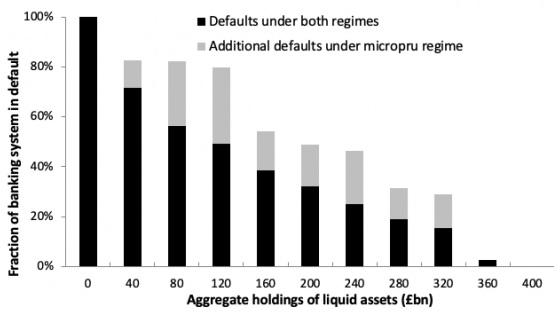
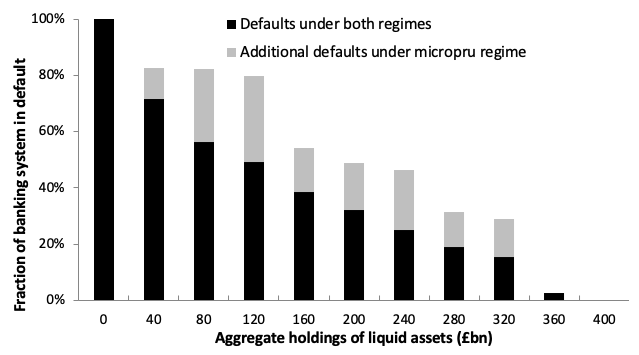
In this setting, the fraction of the banking system in default increases as aggregate liquid asset holdings fall (see Figure 2). As in Aldasoro and Faia (2016), this finding underscores the importance of microprudential liquidity requirements in improving systemic stability. However, these requirements are inefficiently distributed across banks. For example, when banks hold £320 billion of liquid assets, 29% of the banking system would be in default when liquidity requirements are calibrated ‘microprudentially’ – that is, without taking into account externalities and network effects. In a macroprudential regime where liquidity requirements are optimally distributed in the cross-section of banks, this share almost halves to 16%. The macroprudential regime is more efficient because requirements are concentrated in banks whose failure would generate a cascade of non-repayments.
Figure 2 Fraction of the banking system in default by aggregate liquid asset holdings
Source: Ferrara et al. (2019).
Note: The simulation proportionally scales the interbank funding network by 300% of its end-2013 size to mimic the pre-crisis interbank market.
Conclusion
The availability of granular network data allows regulators to differentiate liquidity requirements according to banks’ systemic importance. By skewing liquidity requirements towards systemically important banks, systemic risk can be reduced even when requirements for less important banks are relaxed. The novel insight is that a macroprudential approach to liquidity requirements can enhance financial stability without increasing the aggregate burden of regulation.
Authors’ note: The views expressed are those of the authors and do not necessarily represent those of the institutions to which they are affiliated.
References
Aldasoro, I and R Faia (2016), “Systemic loops and liquidity regulation”, Journal of Financial Stability 27: 1-16.
Basel Committee on Banking Supervision (2013), “Basel III: The Liquidity Coverage Ratio and liquidity risk monitoring tools”.
Borio, C (2009), “The macroprudential approach to regulation and supervision”, VoxEU.org, 14 April.
Eisenberg, L and T Noe (2001), “Systemic risk in financial systems”, Management Science 47(2): 236-249.
Farhi, E and J Tirole (2013), “Collective moral hazard, maturity mismatch, and systemic bailouts”, American Economic Review 102(1): 60-93.
Ferrara, G, S Langfield, Z Liu and T Ota (2019), “Systemic illiquidity in the interbank network”, Quantitative Finance 19(11): 1779-1795.
Stein, J (2013), “Liquidity regulation and central banking”, speech at the 2013 Credit Markets Symposium sponsored by the Federal Reserve Bank of Richmond.
Endnotes
[1] Outflows are calculated by multiplying various categories of liabilities, such as deposits, by the rates at which they are expected to run off under stress.