In many situations, decision-makers can choose actions that create both benefits for a small group and large costs that are dispersed among many others. For example, when a politician considers a policy that is favoured by a special interest group, she has to weigh the benefits for this group against the costs that the policy creates for the general public. Or, when a physician determines a patient’s treatment, she affects not only the patient’s wellbeing but also the treatment costs that the insurance company (and hence the group of customers of this company) or the taxpayer has to bear as a result (Bertola and Koeniger 2011). To analyse these choices, economists need to know the extent to which people take dispersed costs into account.
In a new study, we use experimental games to examine distributional preferences in situations that entail concentrated benefits and dispersed costs (Schumacher et al. forthcoming). In each game, there are three parties: a decider who chooses the provision of a good, a poor receiver who potentially benefits from the good’s provision, and a number of payers who bear the costs of provision. What matters for decision-making in these situations is the extent to which the decider takes the number of payers into account. An individual who cares about equality may equalise the receiver’s and a payers’ payoff, even if the benefits for the receiver are much smaller than the total costs for the payers. Similarly, a selfish individual may choose to maximise her own payoff, regardless of the number of payers. We say that such individuals are ‘insensitive to group size’.
Individuals who are insensitive to group size may display strange behaviours. They may behave generously when sharing an endowment with a poor person, but also take from many others when the costs per affected person is small, or they may care for efficiency when resources are redistributed between two individuals, but also ignore the costs of redistribution if these costs are sufficiently dispersed.
Study design
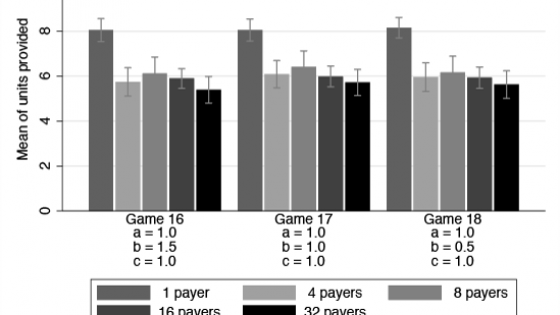
In our experiment, we vary the number of payers to estimate the share of subjects who are insensitive to group size. Consider Figure 1 below. It displays the provision of the good in three situations where the decider earns amount a per unit provided, the receiver earns b and each payer pays c. We have five treatments with either 1, 4, 8, 16, or 32 payers. As can be seen, deciders react to the number of payers when we have four payers instead of one – the provisions of the good then drops significantly. However, when we increase the number of payers even further, on average they make the same choices irrespectively of whether there are 4, 8, 16 or 32 payers. In treatments with large numbers of payers, these choices reduce the group payoff considerably. For example, in Game 17 below, where both the decider and the receiver earn 1 per unit provided, and each payer pays 1 per unit provided, the average decision is to provide 5.6 units. This creates total benefits of 11.2 for the decider and the receiver, but also total costs of 179.2 for the payers – an enormous waste. The question is whether these choices can be explained by selfishness, the desire to equalise payoffs, or an insensitivity to group size.
Figure 1 Average payoffs for groups of 1, 4, 8, 16 and 32 payers

We test individuals’ behaviour in a sequence of decision-making situations in which only the decider or only the receiver benefits from the provision of the good. Through this design we can disentangle the different behavioural motives. We find that around 40% of individuals are not completely selfish, but insensitive to group size when only the decider benefits. Roughly the same number of individuals are not completely selfish (or only caring for equality), but insensitive to group size when only the decider benefits. These two groups are not identical. The estimated correlation is 0.63 so that around 65% of individuals are insensitive to group size in one way or the other. These individuals take the welfare of all parties into account, but ignore the size of an affected group.
We also observe the types of ‘strange behaviours’ we described above. About a quarter of those who behave most generously in simple dictator games (in which they share their endowment with the poor receiver) also take the maximal amount from the payers once costs are large and dispersed. And those with the highest degree of efficiency concerns in dictator games just behave like everybody else in situations with concentrated benefits and dispersed costs.
Potential explanations
One potential explanation for these results is the human ability to put oneself in someone else’s position. In The Theory of Moral Sentiments, Adam Smith proposed that humans have a strong tendency and ability to empathise with others (Smith 1759). Yet, it seems difficult to put oneself in the position of a whole group of people. The more natural thing would be to take the position of a representative member of the group, which may induce decision-makers to ignore the size of an affected group. Consistent with this, Kahneman et al. (1999) argue that individuals value groups of people ‘by prototype’ – an individual’s attitudes towards a group are primarily determined by her attitudes towards a representative member of this group, implying that group size receives only little weight in the valuation.
Implications
Our findings provide a new explanation for a number of important empirical patterns. First, they suggest that many people may approve of political or economic decisions that create benefits for a few but at the same time entail large costs for society (such as collective action of small occupation groups). A classic argument in the literature is that small groups are more successful in achieving their common interests through lobbying than large groups, because they are better able to overcome the free-rider problem (Olson 1965). Our results indicate that unaffected third parties (such as politicians, judges, or voters) may already be positively inclined towards policies with concentrated benefits and large, dispersed costs. Thus, lobbying might not even be necessary to implement them.
Second, our results imply that both pro- and antisocial decisions can be individually optimal at the same time. Take, for example, donations to a needy person and tax evasion. The decider maximises utility from both activities, possibly at different points in time and with a different mindset, but with the same distributional preferences. If the decider is altruistic towards the receiver, she donates a positive amount. If, additionally, she is insensitive to group size and the number of affected citizens is large, she also evades taxes. Thus, optimal levels of donations and hidden income can be strictly positive at the same time.
Third, a physician who is insensitive to group size may have concerns for the patient, but not for the group of insurance payers. Thus, she may recommend an expensive but inessential treatment to patients who are insured, but not to those who pay for themselves. This mechanism may contribute to growth in health care costs (e.g. Chandra and Skinner 2012).
Finally, social preferences with insensitivity to group size imply that charity donations to a single individual can exceed those to a large group. Indeed, Kogut and Ritov (2005) find that contributions for a single victim exceed those for a group of eight victims when these two situations are judged separately. Hence, to maximise a giver’s propensity to donate it is important for charity organisations to highlight the fate of one specific recipient that depends on the giver’s benevolence.
References
Bertola, G, and w Koeniger (2011), “What determines the optimal mix of public and private insurance?”, VoxEU.org
Chandra, A, and J Skinner (2012), “Technology Growth and Expenditure Growth in Health Care”, Journal of Economic Literature, 50 (3), 645-680
Kahneman, D, I Ritov, and D Schkade (1999), “Economic Preferences or Attitude Expressions?: An Analysis of Dollar Responses to Public Issues”, Journal of Risk and Uncertainty, 19 (1), 203-235
Kogut, T, and I Ritov (2005), “The Singularity Effect of Identified Victims in Separate and Joint Evaluations”, Organizational Behavior and Human Decision Processes, 97 (2), 106-116
Olson, M (1965), The Logic of Collective Action: Public Goods and the Theory of Groups, Harvard University Press
Schumacher, H, I Kesternich, M Kosfeld, and J Winter (forthcoming), “One, Two, Many – Insensitivity to Group Size in Games with Concentrated Benefits and Dispersed Costs”, Review of Economic Studies
Smith, A (1759), The Theory of Modern Sentiments.