How should the government target student financial aid? Programmes like the Pell Grant in the US provide need-based financial aid, which is spending targeted at underprivileged students. This reduces inequality by providing aid to low-income families, and should lead to an increase in social mobility (Black et al. 2016). But is such a policy optimal from an efficiency point of view?
In a recent paper (Colas et al. 2020), we combine theoretical and empirical analyses to study how financial aid schedules should optimally vary with parental income. We show that optimal financial aid should be highly need-based, much more so than the current financial aid system in the US. This result is not driven by a desire to reduce inequality, but instead is based on the grounds of efficiency: providing financial aid to children whose parents have weak financial backgrounds implies a much higher return on each dollar spent. Subsidising college for children with low family incomes is a great investment in future tax revenue. Need-based financial aid both reduces inequality and is optimal from an efficiency point of view. In this case, equity and efficiency go hand in hand.
Theory
We begin by solving for the optimal schedule in a general life-cycle model. Agents differ in multiple dimensions, including ability and parental income. Individuals decide whether to enrol in college or enter the labour market directly after high school. We also model the decision to drop out or stay enrolled and finally graduate. The lifecycle process of wages, which varies with educational decisions and ability, is modelled in detail. We derive a simple optimality condition for how financial aid should vary with parental income. The formula transparently highlights key trade-offs.
The three main statistics that enter the equation are as follows. First, the government should optimally provide financial aid to groups with many ‘marginal’ members (i.e. individuals on the verge of attending college such that a small increase in financial aid would induce them to attend). Targeting financial aid towards groups with many marginal students will lead to large increases in enrolment. Second, we show that the government should target aid towards individuals whose college attendance will lead to large social benefits. This is captured by the ‘fiscal externality’ of marginal individuals (i.e. the amount an individual’s lifetime tax payments will increase as a result of attending college). Third, optimal financial aid decreases in the share of college enrolees, whom we refer to as ‘inframarginal’ students. A higher share of inframarginal students implies a higher marginal cost of increasing financial aid; if the planner increases financial aid by $1, these inframarginal students must all be paid an additional dollar without any effect on their enrolment decisions.
Whether it is optimal to have need-based financial aid (i.e. financial aid that decreases as parental income rises) depends on how these three statistics vary with parental income. An important consideration is that these three statistics are not policy invariant. As we move from current to optimal policies, these statistics will change. To quantitatively characterise the optimal financial aid schedule for the US, we therefore estimate a fully specified structural model.
Structural model
We estimate a rich structural model of college attendance that allows us to compute these statistics along the parental income distribution and for alternative policies to solve for the optimal financial aid schedule. In our quantitative structural model, we account for earnings risk, dropout, labour supply during college and, importantly, crowding-out of parental transfers. To capture the latter, we explicitly model parental decisions to save, consume, and provide transfers to their children. Another crucial ingredient of the model is heterogeneity in the psychic costs of education, because monetary returns account for only a small part of observed college attendance patterns (Heckman et al. 2006). Using data from the National Longitudinal Survey of Youth from 1979 and 1997, we estimate the parameters of our model via maximum likelihood.
The model successfully replicates quasi-experimental studies. First, it is consistent with estimated elasticities of college attendance and graduation rates with respect to financial aid expansions (Deming and Dynarski 2009). Second, it is consistent with the causal impact of parental income changes on college graduation rates (Hilger 2016). Further, our model yields (marginal) returns to college that are in line with the empirical literature (Card 1999, Oreopoulos and Petronijevic 2013, Zimmerman 2014).
Quantitative results
We then use the estimated structural model to solve for the optimal financial aid schedule. The policy experiment is such that the government can target financial aid to students with different incomes but is not allowed to increase taxes. We simulate the optimal budget-neutral financial aid reform and consider a dynamic net-present-value budget constraint. Note that a change in the financial aid schedule changes the size and composition of the set of individuals attending college. This implies a change in tax revenue and transfer spending that directly feeds back into the available resource for financial aid.
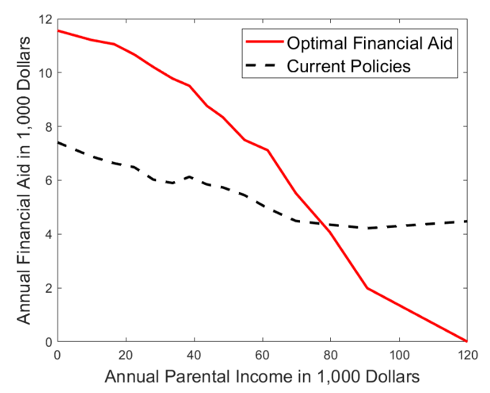
The results for the optimal financial aid schedule are shown in Figure 1. We can see that the optimal aid schedule is highly need-based, as the level of financial aid drops by 48% moving from the 25th percentile of the parental income distribution to the 75th percentile. This is considerably more progressive than the current financial aid system in the US.
Figure 1 Optimal need-based financial aid and current policies
Notes: The solid red line shows the optimal need-based financial aid schedule as a function of parental income. The dotted black line shows the current financial aid schedule, as estimated in the NLSY97.
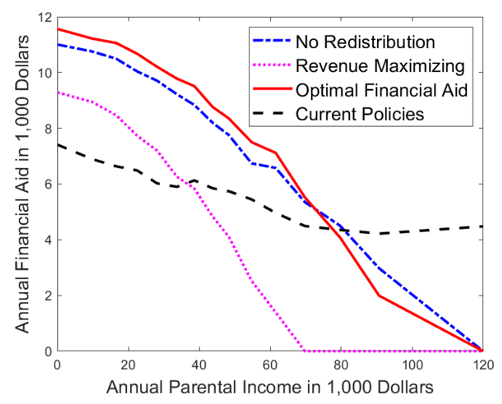
One might be suspicious of whether the progressivity is driven by a desire for redistribution from rich to poor students, given that we employ a utilitarian welfare function. We therefore consider two alternative social planners who do not value redistribution: a social planner who sets equal marginal social welfare weights (Saez and Stantcheva 2016) on all students, and a planner who is only interested in maximising tax revenues. The results are displayed in Figure 2. Both would choose an almost equally progressive financial aid schedule. These results suggest that financial aid policies for students are a rare case in which there is no equity-efficiency trade-off.
Figure 2 Financial aid policies with no redistribution motive
Notes: The solid red line shows the optimal need-based financial aid schedule as a function of parental income. The dashed black line shows the current financial aid schedule, as estimated in the NLSY97. The dashed-dotted blue line shows the optimal schedule for a social planner with no redistribution motive. The dotted magenta line shows the optimal schedule under the objective of maximising net-tax revenue (net of expenditures for financial aid).
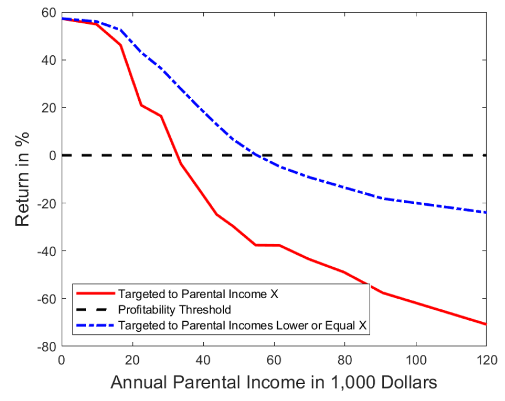
Finally, in Figure 3, we show that an increase in financial aid can be self-financing if properly targeted. The solid red line in Figure 3 illustrates the fiscal return; that is, the net effect on government revenue were financial aid for a particular income level to be increased by $1. For example, a 40% return implies that the net present value increase in tax revenue is 40% larger than the cost of increasing financial aid. Returns are positive for parental income between $0 and $33,000; the latter number corresponds to the 32nd percentile of the parental income distribution. This result is striking: increasing subsidies for this group is a ‘free lunch’. An alternative would be to consider reforms where financial aid is increased for students below a certain parental income level. This case is illustrated by the dashed-dotted blue line in Figure 3. An increase in financial aid targeted to children with parental income below $54,000 is slightly above the margin of being self-financing.
Figure 3 Fiscal returns on increase in financial aid
Notes: The dashed-dotted (blue) line shows the net fiscal return for a $1 increase in financial aid targeted to all students with a parental income level lower than X. The solid (red) line shows the net fiscal return for a $1 increase in financial aid targeted to all students with a parental income level equal to X.
Conclusion
We find that a cost-effective targeting of financial aid goes hand in hand with goals of social mobility and redistribution. This result holds for different social welfare functions, assumptions on credit markets for students, and assumptions on income taxation. Moreover, we find that a progressive expansion in financial aid policies could be self-financing through higher tax revenue, thus benefiting all taxpayers as well as low-income students directly. It seems that financial aid policies are a rare case with no classic equity-efficiency trade-off. We think that our results can be used for policy recommendations according to the criteria of Diamond and Saez (2011). The economic mechanism is empirically relevant and of first-order importance to the problem, it is very robust, and progressive financial aid systems are clearly implementable as they are universal across all OECD countries.
References
Black, S, A Filipek, J Furman, L Giuliano and A Narayan (2016), “Student loans and college quality: Effects on borrowers and the economy”, VoxEU.org, 4 August.
Card, D (1999), “The Causal Effect of Education on Earnings”, Handbook of Labor Economics 3: 1801–1863.
Colas, M, S Findeisen and D Sachs (2020), “Optimal Need-Based Financial Aid”, Journal of Political Economy (forthcoming)
Deming, D and S Dynarski (2009), “Into College, Out of Poverty? Policies to Increase the Postsecondary Attainment of the Poor”, NBER Working Paper 15387.
Diamond, P A and E Saez (2011), “The Case for a Progressive Tax: From Basic Research to Policy Recommendations”, Journal of Economic Perspectives 25: 165–190.
Heckman, J J, L J Lochner and P E Todd (2006), “Earnings Functions, Rates of Return and Treatment Effects: The Mincer Equation and Beyond”, Handbook of the Economics of Education 1: 307–458.
Hilger, N G (2016), “Parental Job Loss and Children's Long-Term Outcomes: Evidence from 7 Million Fathers’ Layoffs”, American Economic Journal: Applied Economics 8: 247–283.
Oreopoulos, P and U Petronijevic (2013), “Making College Worth It: A Review of Research on the Returns to Higher Education”, NBER Working Paper 19053.
Saez, E and S Stantcheva (2016), “Generalized Social Marginal Welfare Weights for Optimal Tax Theory”, American Economic Review 106: 24–45.
Zimmerman, S (2014), “The Returns to College Admission for Academically Marginal Students”, Journal of Labor Economics 32: 711–754.