In representative democracies, the few decide on behalf of the many. But how few? This is not a new problem. The appropriate “representation ratio” was discussed by the founding fathers of the American constitution. In the Federalist Papers, James Madison wrote:
“However small the Republic may be, the Representatives must be raised to a certain number, in order to guard against the cabals of a few; and however large it may be, they must be divided to certain number, in order to guard against the confusion of a multitude” (Federalist Paper n° 10)
In a recent research paper,1 we have derived a theory – a square-root “formula” – for the optimal number of representatives. We have then studied how this formula fits the data on populations and parliaments in a sample of more than a hundred countries. We found a surprisingly good fit. There are some “outliers”, i.e. countries for which our “formula” does not fit well, and we find that those with an excess number of representatives tend to be plagued by red tape, state interference and corruption.
In a nutshell, a parliament with too few representatives is not “democratic” enough, possibly leading to an unstable political system, in which various undesirable forms of political expression, including of course violent ones, will develop. In contrast, too many representatives entail substantial direct and indirect social costs, they tend to vote too many acts, interfere too much with the operation of markets, increase red tape and create many opportunities for influence, rent-seeking activities and corruption.
Our theory is essentially based on a statistical trade-off. Having more Members of Parliament (MEPs) improves the likelihood that a set of randomly-selected MEPs accurately reproduces the population’s preferences. Having more MEPs, however, generates direct and opportunity costs. The optimal number of representatives equalises the social value of an additional seat in parliament, which stems from a reduction of the errors made in the estimation of citizens’ tastes, and the social cost of this additional seat. The computation is made a bit more complicated than it seems at first glance because, in theory, representatives must be provided with incentives not to distort the revelation of preferences. In other words, we worry about the incentives facing MEPs; we do not assume that representatives are benevolent. As it turns out, this consideration is of secondary importance.
Under these assumptions, we show that the optimal number of representatives in a given country should be proportional to the square root of the population. The factor of proportionality decreases with higher costs of representation and is increasing in measures of the country’s dispersion of preferences. The theory should not be interpreted too literally. It says that the optimal number of seats in parliament is given by an increasing, concave, banana-shaped curve plotting the count of representatives against population size. Real-world data will not fit exactly since other factors affect the proportionality factor between the number of representatives and the square root of population size – not all of which are observed by the econometrician.
Not Penrose’s Rule
It is worth mentioning that our “square-root” theory has no connection with Penrose’s “square-root law” of fair representation, which recently attracted some attention during the debates surrounding the Nice Treaty, the Constitutional Treaty.2 Penrose’s law is a way of solving the vote-apportionment problem in a weighted voting system like the EU’s qualified majority voting. In contrast, our banana-shaped curve gives the optimal total number of seats in the representative institution itself, under the assumption that each representative has one vote and the majority threshold is the standard 50%.
Reality check
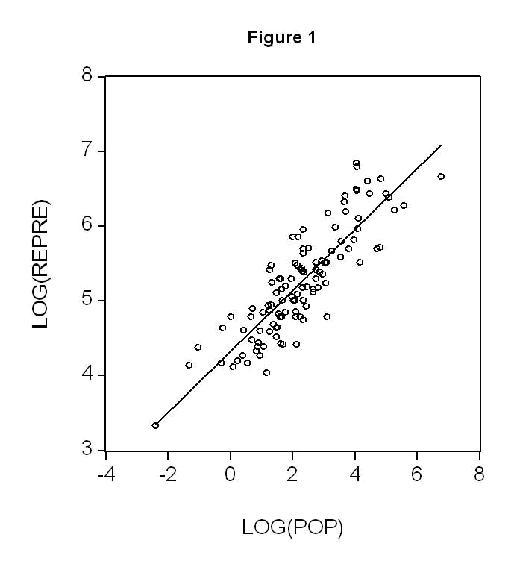
If our theory is right and nations design their democracies with efficiency, the actual numbers of representatives in nations should roughly fit our prediction. To confront the theory with the data, we have run a number of tests on a sample of 111 countries in the year 1995. The results show that the number of representatives is nearly proportional to total population raised to the power of 0.4. Of course, 0.4 is not ½ so the best fit is not the square-root of total population; it is a somewhat smaller power. The precision and robustness of the estimates are fairly good (as can be seen on figure 1).
On Figure 1, the logarithm of the number of representatives is plotted against the logarithm of the country’s population (in millions), along with the least squares regression line. The theoretical banana-shaped curve should appear as a straight line (with slope ½) on the figure. The slope is in fact close to 2/5.
If we take the “N0.4 model” as the fitted value, most countries lie within a reasonable distance of this worldwide benchmark. There are also obvious outliers, that is, countries with abnormally high or low numbers of representatives (in relative terms). We can distinguish five groups.
- The countries with an abnormally high number of seats in national parliament: France, Italy and Spain. With 898 seats in the National Assembly and the Senate taken together, France has more representatives than the United States (again adding the House and the Senate together). According to our computations, France’s optimal number is 545. Italy’s optimal number is 570, but this country has a total of 945 representatives in 1995.
- The countries with too many, albeit not a plethoric number of seats. This group includes Greece, Switzerland, Ireland and the UK (if we put the Peers aside in this latter case, and count only the MPs).
- The “good guys”, that lie more or less on the banana-shaped curve; this group includes some heavyweights: Canada, Germany, Finland, India, Japan, Portugal, Russia and Sweden.
- The group with (moderately) too few representatives: Austria, Australia, Belgium, Denmark and Norway. We invite the reader consider whether these countries have something else in common.
- The nations with abnormally sub-optimal representations: Israel, New Zealand, the Netherlands and above all, the USA. Nations in the last group are all close to a ratio of 65% of their optimal representation level. The US has 535 national representatives (if we add the House of Representatives and the Senate), but our model predicts that the Congress should have 807 seats instead. In America, a high degree of institutional rigidity seems to be the cause of the insufficient representation: the number of US representatives has been fixed by statute in 1929, and the number of voters per representative has constantly increased, through the entire US history, to reach record highs in the recent years.
The cost of deviation for the benchmark
If our theory is right, there should be a cost to nations that have too few or too many representatives. The cost from having too few is difficult to observe. In our theory, having too few representatives makes it likely that the democracy will not be sufficiently representative. Testing for this, however, would require detailed knowledge of the choices of the nations and the preferences of the population. It is easier to observe the problems of too many representatives. According to our theory, such nations should experience extra social costs.
Our preliminary study of the facts shows that a country’s excess number of representatives is significantly correlated with more red tape (a measure of the direct cost of meeting the requirements to open a new business), more state interference (a measure of whether state interference hinders business development) and more perceived corruption (as measured by Transparency International’s well-known corruption index).
Why is that true? We suggest what might be called a “quantity theory” of legislation; more lawmakers mean more law, i.e. more rules and regulations. These new rules, in turn, tend to interfere with the operation of free markets as they are motivated by the desire, for example, to protect pressure groups and various lobbies from competition. At the same time, regulations tend to create temptations and opportunities for more influence activities, cronyism, bribes, capture, and sheer corruption.
Our ideas and observations are still exploratory, and further work is of course needed to confirm the validity and robustness of these findings. Yet, our results strongly suggest that some countries, such as France or Italy, could reconsider the appropriateness and cost-efficiency of their political institutions. Another interesting question is the optimal number of Euro MPs. The total population of the EU is now 490 million; there are currently 785 euro representatives, and according to our computations, the optimal number of seats should now be roughly equal to 890.
Footnotes:
1 The classic work on these questions is J. Buchanan and G. Tullock (1962), “The calculus of consent”, University of Michigan Press. On voting theory, see Austen-Smith and Banks (1999), “Positive Political Theory”, University of Michigan Press; see also H. Moulin (1988), Axioms of Cooperative Decision-Making, Cambridge University Press. On recent developments of the “Public Choice” literature, see Mueller (2003), “Public Choice III”, Cambridge University Press. Our own paper is available at http://www.cepr.org/pubs/new-dps/dplist.asp?dpno=6417; as far as we know, our argument for the “square-root formula”, based on Mechanism Design, is new.
2 It was advocated Sweden in the IGC2000 leading up to the Nice Treaty and Poland last year in the negotiations of the Reform Treaty; see http://voxeu.org/index.php?q=node/262 on the logic of Penrose’s rule.