Ever since Harris and Gurel (1986) and Shleifer (1986) documented that when stocks are added to the S&P 500 index their prices rise, economists have been debating what to make of this result. There is now a large literature confirming these findings and many theories that aim to explain them. Yet almost all of the subsequent research has focused on how indices influence asset prices, with much less attention paid to other possible implications. In a recent paper (Kashyap et al. 2018), we look at the repercussions of index inclusion for corporate decisions.
Asset management practices affect corporate decisions
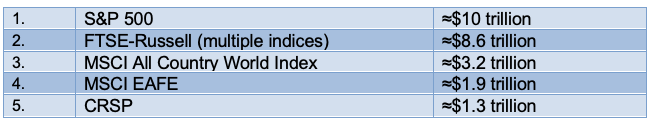
The asset management industry is estimated to control more than $85 trillion worldwide. Most of this money is managed against benchmark indices. For example, just under $10 trillion is benchmarked to the S&P 500 alone. Table 1 lists other prominent benchmarks. We argue that firms included in a benchmark are effectively subsidised by asset managers and so should evaluate investment opportunities differently.
Table 1 Money managed against leading benchmarks
Notes: The figures for the S&P 500 and MSCI indices are as of the end of 2017, for FTSE-Russell as of the end of 2016 and for CRSP as of September 2018.
We construct a model with asset managers to demonstrate that firms that are part of a benchmark have a different cost of capital than similar firms outside the benchmark. When a firm adds risky cash flows, say, because of an acquisition or by investing in a new project, the increase in the shareholder value is larger if the firm is inside the benchmark. Hence, a firm in the benchmark could accept a project that an otherwise identical non-benchmark firm would not. This runs contrary to standard corporate finance theory. In the special case where there are no asset managers – as is standard in corporate finance – investors always value equivalent cash flows of benchmark and non-benchmark firms in the same way.
The underlying reason for why benchmark inclusion affects firms’ investment decisions is the performance evaluation of asset managers. In keeping with prevalent industry practices and academic evidence such as Ma et al. (2019), in our model an asset manager’s compensation depends on her relative performance compared to a benchmark index.
We show that the asset manager’s optimal portfolio is a combination of the usual mean-variance portfolio and the benchmark portfolio – the latter appearing because of the relative performance considerations. Underperformance relative to the benchmark is an additional source of risk for the asset manager and she hedges this risk by holding a fixed fraction of her portfolio in benchmark stocks. She does so regardless of the benchmark stocks’ prices and characteristics of their cash flows – in particular, irrespective of cash-flow variance. An extreme special case of our model is when our asset managers are passive. In that case they invest their entire portfolio in the benchmark, regardless of its risk-return trade-off.
When a firm inside the benchmark adds risky cash flows (e.g. invests in a project), asset managers buy more claims to these cash flows than to equivalent cash flows of a peer that is outside the benchmark. The increase in the shareholder value is thus higher if the firm is inside the benchmark rather than outside. We call this the ‘benchmark inclusion subsidy’.
For instance, consider a benchmark and a peer non-benchmark firm contemplating investing in a risky project. When the benchmark firm invests, the extra variance of its cash flows resulting from the project will be penalised less than that of an identical non-benchmark firm. Why? The reason is the mechanical demand of asset managers for stocks inside their benchmarks, regardless of the variance. Thus investing in a project increases the firm’s stock value by more if the firm is in the benchmark. Put differently, investment is effectively subsidised for the benchmark firm. Because the subsidy is tied to cash-flow risk, however, the two firms will still value risk-free projects identically.
We have specific cross-sectional predictions for the size of the benchmark inclusion subsidy. We show that the higher the cash-flow risk of an investment, the larger the benchmark inclusion subsidy. Moreover, the size of the subsidy rises as the asset management sector grows in size. Finally, the larger is the fraction of assets managed by passive managers, the larger the subsidy. The latter implication is due to the fact that passive managers invest their entire portfolio in the benchmark while active managers split it between the mean-variance portfolio and the benchmark.
Adding correlations
The results described so far do not require any correlation between a new investment (or acquisition) and the firm’s assets in place. Allowing for correlations brings out additional effects and predictions. As before, asset managers' excess demand for benchmark stocks raises those stocks' prices. Now, the stock prices of firms whose cash flows are correlated with those of the benchmark stocks also rise. This happens because, in seeking exposure to the benchmark's cash-flow risk, investors substitute away from expensive benchmark stocks into stocks that are positively correlated with them. This same reasoning means that new investment projects or acquisitions that are positively correlated with the benchmark should be valued more by all firms, both inside and outside the benchmark.
We derive the precise expression for the benchmark inclusion subsidy for the case with correlated cash flows and show that, by selecting a project that is positively correlated with its assets-in-place, a benchmark firm can increase the benchmark inclusion subsidy further. The subsidy is the largest for projects that are clones of a firm’s existing assets. As the correlation of a project’s cash flows with the existing assets drops, so does the benchmark inclusion subsidy.
Our model also implies that the right model for evaluating the cost of capital in our environment is not the standard capital asset pricing model (CAPM), but its two-factor modification that accounts for the presence of asset managers. The CAPM continues to be the leading model for the computation of the cost of capital in practice. As the asset management industry continues to grow, perhaps it is time to rethink this.
The magnitude of the cost of capital reduction
Our estimates of the reduction of the cost of capital owing to a benchmark inclusion range from 30 basis points to a full percentage point. To get a feel for the magnitude, one can compare it to a typical loosening of 25 basis points by a central bank. Our estimates of the magnitudes are model-implied. Interestingly, a recent empirical analysis by Calomiris et al. (2018) reports magnitudes within the same range as ours for the reduction in the cost of borrowing of firms in emerging markets if they issue index-eligible bonds.
Suggestive empirical evidence
We put together a host of empirical evidence consistent with the implications of our model. Bena et al. (2017) study differences in investment and employment for firms across 30 countries between 2001 and 2010. Consistent with our theory, they find a large and statistically significant effect of the benchmark additions on both investment and employment.
Our theory is consistent with the index effect, i.e., the rise (fall) in stock prices upon index inclusion (deletion), which is widely documented in the literature. The estimated magnitudes of the index effect vary across studies, and typically most of the effect is permanent. For example, Chen et al. (2004) find the cumulative abnormal returns of stocks added to the S&P 500 during 1989-2000, measured over two months after the announcement, to be 6.2%.
Chang et al. (2015) study transitions of stocks from the Russell 1000 (top 1000 stocks) to the Russell 2000 (stocks 1001 to 3000) index and vice versa. They look at firms whose fortunes improve, prompting a move from the widely benchmarked Russell 2000 index to the less-benchmarked Russell 1000. The authors find that despite the improved fundamentals, their stock price drops by about 5% from the rebalancing, mirroring the drop in demand by asset managers. Conversely, firms that fall into the Russell 2000 see price increases by about 5%.
We also have a more subtle prediction – the stock price response should depend on becoming part of a benchmark and not just because of being added to an index. It is possible to separate the effect of being in the index from that of the benchmark for firms that operate in so-called ‘sin’ industries, such as alcohol, tobacco and gaming. Large firms in these industries would be included in indices such as the S&P 500, Russell 1000 or FTSE 100, but are screened out of their benchmarks by socially responsible investors. Hong and Kacperczyk (2009) document that sin firms earn higher expected returns than comparable firms by about 2.5% per year and that their valuations relative to matched non-sin firms are 15% to 20% lower.
Conclusions
While there is empirical evidence that speaks to some of our predictions, there are others that have yet to be tested. For example, the literature on index inclusion has only looked at the average effect of inclusion. We have specific cross-sectional predictions for the size of this effect. It would also be interesting to test the model’s predictions about how the presence of benchmarks can alter the incentives regarding mergers.
Asset managers already hold a sizeable fraction of the global stock and bond markets. As the industry continues to grow, the benchmark inclusion subsidy will only get bigger.
References
Bena, J, M Ferreira, P Matos and P Pires (2017), “Are foreign investors locusts? The long-term effects of foreign institutional ownership”, Journal of Financial Economics 126(2): 122-146.
Calomiris C W, M Larrain, S L Schmukler and T Williams (2018), “The search for yield and the size premium in emerging market corporate debt”, Columbia University working paper.
Chang, Y-C, H Hong and I Liskovich (2015), “Regression discontinuity and the price effects of stock market indexing”, Review of Financial Studies 28: 212-246.
Chen, H, G Noronha and V Singal (2004), “The price response to S&P 500 index additions and deletions: Evidence of asymmetry and a new explanation”, Journal of Finance 59: 1901-1929.
Harris, L and E Gurel (1986), “Price and volume effects associated with changes in the S&P 500 list: New evidence for the existence of price pressures”, Journal of Finance 41: 815-829.
Hong, H and M Kacperczyk (2009), “The price of sin: The effects of social norms on markets”, Journal of Financial Economics 93: 15-36.
Kashyap, A, N Kovrijnykh, J Li and A Pavlova (2018), “The benchmark inclusion subsidy”, CEPR discussion paper 13356.
Ma, L, Y Tang and J-P Gómez (2019), “Portfolio manager compensation in the US mutual fund industry”, Journal of Finance, forthcoming.
Shleifer, A (1986), “Do demand curves for stocks slope down?”, Journal of Finance 41: 579-590.