US Treasuries serve a crucial role as global safe assets. Treasury yields are also applied as benchmark long-term interest rates and yield changes around Fed announcements are used to gauge the effects of monetary policy. How are US Treasury yields and returns determined and how do they react to new information?
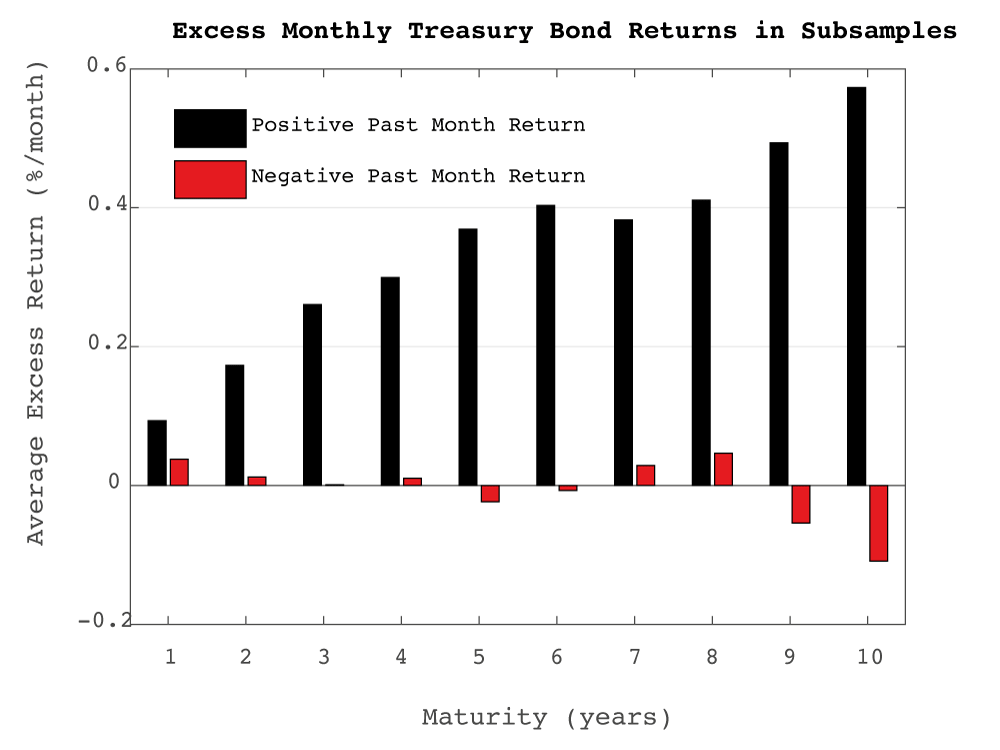
In a recent paper (Sihvonen 2024), I analyse time series momentum along the Treasury term structure. Figure 1 illustrates a striking momentum pattern in the Treasury market: monthly excess bond returns are clearly higher following months with positive rather than negative returns. Returns after months with negative returns are close to zero, so that essentially all of the total bond returns are earned in months in which the past month return was positive. This momentum in returns is caused by autocorrelation in yield changes. A decline in yields, caused for example by a Fed rate drop or a macroeconomic release, tends to be followed by further yield declines in the following month. While the effects on next month returns are strong, the momentum is short-lived with insignificant effects on returns after the next month. This is why the momentum easily gets unnoticed in studies applying annual bond returns.
Figure 1 Excess monthly Treasury bond returns in subsamples
The observed momentum has implications for investment strategies, forecasting yields, measuring announcement effects, as well as theoretical models of the yield curve.
Implications for investment strategies and empirical analysis
First, investors can gain from trend following strategies that overweight long-term Treasuries following months with positive returns. The short-lived nature of momentum implies that, for the Treasury market, such strategies should have both short lookback horizons and holding periods. Still, I demonstrate that the profits from such strategies survive reasonable estimates of transaction costs for professional investors and can also be implemented using futures contracts. Moreover, the momentum is not caused by stale prices, measurement error or similar mechanical issues.
Long-term yields and bond returns are more volatile than those for shorter maturities. This noise means it can be more difficult to detect the momentum for long rather than short maturity bonds. However, the momentum exists and is tradable across the maturity spectrum. Moreover, the strong factor structure in yields, in particular the importance of the level factor, implies that the specific maturity used for calculating the past return or yield change is not critical.
The predictive power of past returns also survives after controlling for yields and macroeconomic variables. This implies that optimal short-term forecasts of Treasury bond returns and yields should account for past returns. However, while accounting for past returns or yield changes clearly improves short-term yield forecasts, the relative effects on forecasts several years ahead are smaller.
The final empirical implication concerns measuring the effects of a monetary policy or news announcement on Treasury yields. Standard high-frequency identification is based on measuring yield changes during short windows over announcements (e.g. van den End and Samarina 2021, Hanson and Stein 2015.). The benefit of using such short time frames is that the results are unlikely to be contaminated by secondary news events. However, such identification implicitly assumes that all information is swiftly incorporated in the yields. The observed yield drifts after announcements imply that while such identification typically results in the right sign, the magnitudes of the yield changes should be interpreted with caution.
Problems for theoretical models
The above results are inconsistent with standard macro-finance models. This is due to two reasons. First, while such models can generate some momentum through risk effects, this model-implied momentum tends to be clearly smaller than that observed in the data.
Second, and as mentioned, the predictive power of past returns or yield changes survives after controlling for current yields – that is, past returns predict future returns but are unspanned by information in current yields. On the other hand, standard macro-finance models imply full spanning. In particular, and in contrast to the empirical results, these models predict that the predictive power of past returns or yield changes does not survive after controlling for current yields.
Standard behavioural models that relax full information rational expectations can address the first point but not the second. These models can generate quantitatively realistic degrees of momentum through underreaction or sticky expectations. However, the models still imply full spanning so that the momentum should vanish after controlling for yields – that is, the models generate momentum through autocorrelation in yield curve factors, while in the data momentum is caused by a factor unspanned by the yield curve factors.
In my paper I offer a potential theoretical explanation for momentum that addresses both points. In the model agents face complexity constraints and price bonds using a simplified representation of the world. This complexity constraint leads agents to ignore longer-term dependencies in key factors driving bond prices. The difference between the subjectively perceived and actual factor dynamics generates momentum. Moreover, the omitted longer-term dependencies also generate a violation of full spanning.
References
van den End, J W and A Samarina (2021), “Financial market sensitivity to announcements by the ECB”, VoxEU.org, 2 January.
Hanson, S and J C Stein (2015), "Monetary Policy and Long-Term Real Rates”, Journal of Financial Economics 115(3): 429-448.
Sihvonen, M (2024), “Yield Curve Momentum”, Review of Finance, forthcoming.