A notable collection of recent research has described the significant concentration of income and wealth that exists in many countries of the world. According to Atkinson et al (2011) and Wolff (2012), the richest 1% of households in the United States hold more than 33% of total wealth and earn nearly 25% of total income, with similar numbers observed in other countries as well. Díaz-Giménez et al (2002) document that the Gini coefficients of the distributions of income and wealth in the United States are equal to 0.55 and 0.80, respectively, and Davies et al (2011) find similar Gini coefficients for wealth throughout the world.
The protests in Western countries suggest that it is not just academic economists who get this point.
The role of uninsurable risk
Macroeconomists have adopted a number of different approaches to account for the high degree of inequality (see Cagetti and DeNardi 2008 for a recent survey). One of the most recent focuses on the role of uninsurable investment risk and the multiplicative process of wealth accumulation (Benhabib et al 2011). This approach is motivated by the empirical findings of Case and Shiller (1989), Flavin and Yamashita (2002), and Moskowitz and Vissing-Jorgensen (2002). These authors show that households hold significant fractions of their total wealth in the form of principal residence ownership and private business equity, both of which are volatile sources of idiosyncratic investment risk that are not easily diversified away.
In new research (Fernholz and Fernholz 2012), we embrace the insights from this empirical literature and analyse an economy in which households that are identical with regard to patience and skill face uninsurable investment risk, and there is neither explicit redistribution in the form of government tax or fiscal policies nor implicit redistribution in the form of limited intergenerational transfers of wealth.
In this setting, we show that in the absence of redistributive mechanisms, the distribution of wealth is unstable and becomes increasingly concentrated over time until virtually all wealth is held by a single household.
It is important to emphasise that the households in our setup are identical in terms of their patience (preference for consumption today versus consumption tomorrow) and their skill (how much they earn from labour income and how well they are able to invest on average), so the playing field is as level as possible, and it is luck alone – in the form of high realised random investment returns – that generates this extreme divergence.
This result may seem counterintuitive. It relies on results from mathematical finance (Fernholz 2002) that show that at a given moment in time, the growth rate of the total wealth of an economy composed of many households is greater than the average growth rate of the wealth held by each of the individual households. Over the longer term, however, the total wealth of the economy cannot grow faster than the wealth held by every one of its component households. Therefore, over time, the spread of wealth across the households must continually decrease until eventually all the wealth in the economy concentrates with a single household, and in this manner the growth rate of the economy tends to the growth rate of that single household.
Wealth dynamics over time
It is useful to parameterise our model and simulate these dynamics. Our approach is simple enough that the behaviour of the economy and the distribution of wealth over time depend entirely on just one factor: the extent of households' exposure to uninsurable idiosyncratic investment risk. The higher this exposure, the faster the pace at which the economy's total wealth concentrates.
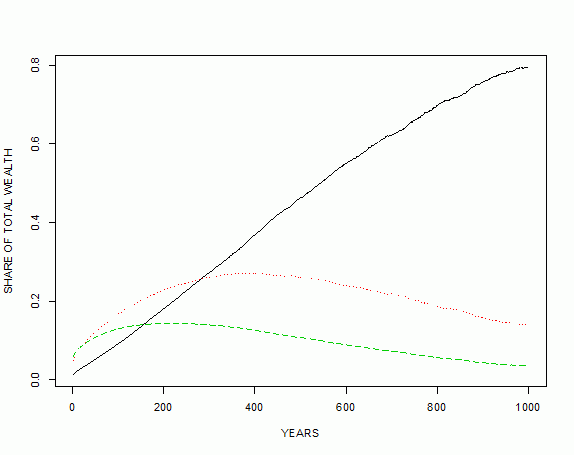
In Figure 1, we plot the shares of the economy's total wealth among one million households under the assumption that households' exposure to uninsurable investment risk, denoted by the parameter σ, corresponds to a 10% standard deviation in yearly investment returns, which is roughly consistent with empirical data. The share of total wealth held by the wealthiest 1% of households is represented by the solid black line, that held by the wealthiest 1%–5% by the dotted red line, and that held by the wealthiest 5%–10% by the dashed green line. Starting from a position in which all households hold the same amount of wealth, the economy gradually evolves so that within 500 years approximately half of the economy's total wealth is held by the wealthiest 1% of households, and after 1,000 years that share increases to nearly 80%. Within 300 years, the economy reaches a position that resembles the United States in 2001 – the wealthiest 1% of households hold 33% of total wealth, while the wealthiest 1–5% and 5–10% of households hold, respectively, 26% and 12% of total wealth.
Figure 1. Shares of wealth over time
Note: The shares of wealth held by the wealthiest 1% (solid black line), the wealthiest 1–5% (dotted red line), and the wealthiest 5–10% (dashed green line) (σ = 0.1).
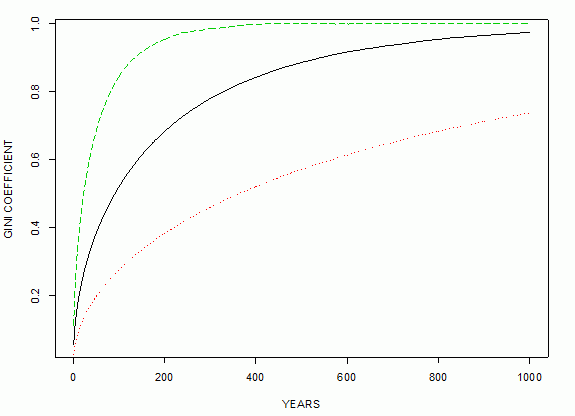
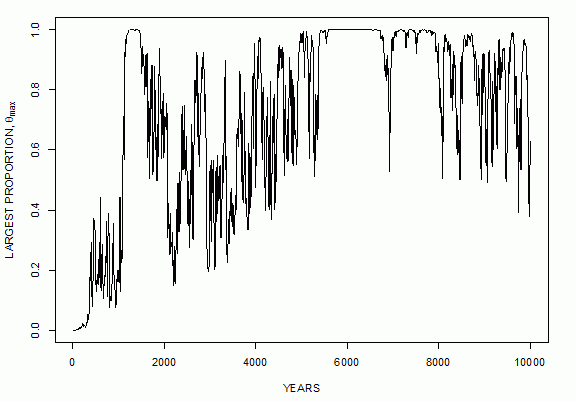
In Figure 2, we vary households' exposure to uninsurable investment risk and plot the evolution of the Gini coefficient over time. The Figure includes the previous parameterisation in which there is a 10% standard deviation in yearly investment returns, and it also includes parameterisations corresponding to 5% (σ = 0.05, represented by the dotted red line) and 20% (σ = 0.2, represented by the dashed green line) standard deviations. The Figure clearly shows that as households' exposure to idiosyncratic investment risk increases, so does the pace at which the economy's wealth concentrates. Finally, in Figure 3 we plot the share of total wealth held by the single wealthiest household in the economy. Although it takes a long time, the Figure demonstrates that the economy's total wealth gradually but steadily concentrates at the top.
Figure 2. Gini coefficients over time
Note: The Gini coefficient of the economy for σ = 0.1 (solid black line), σ = 0.05 (dotted red line), and σ = 0.2 (dashed green line).
Figure 3. Total wealth held by the wealthiest household
Note: The share of total wealth held by the wealthiest single household, (σ = 0.2).
Redistribution and stability
Our analysis highlights the crucial role of redistributive mechanisms in an economy. Indeed, it is their presence alone that ensures the stability of the economy and prevents an outcome in which the distribution of wealth becomes increasingly skewed towards the top.
We interpret redistribution broadly, so that redistributive mechanisms include any process that proportionally affects wealthy households and poor households differently.
Redistributive mechanisms include explicit mechanisms such as government tax or fiscal policies that directly transfer income from wealthy to poor households as well as implicit mechanisms such as limited intergenerational transfers of wealth that reduce the total wealth held by wealthy households proportionally more than that of poor households. Although implicit redistributive mechanisms do not involve direct transfers of wealth from wealthy to poor households, their stabilising effect on the equilibrium distribution of wealth is the same.
Implications and questions
The main conclusion of our analysis is clear. In the absence of any redistribution, the distribution of wealth is unstable over time and becomes concentrated entirely at the top. This occurs despite the fact that all households have identical patience and skill. It is important to emphasise that the setup we analyse, in which there is absolutely no redistribution, is intended to describe a benchmark case rather than to capture the true state of the world. In reality, a number of potentially redistributive mechanisms, such as government tax and fiscal policies and intergenerational transfers, constantly affect the economy and influence the distribution of wealth. Recent work by Gabaix (2009) and Benhabib et al (2011) examines settings in which redistributive mechanisms of this kind are present, and these authors show that this results in stable and empirically realistic Pareto distributions for wealth. Our results complement this research and highlight the stabilising role of redistributive mechanisms in the economy.
There are a number of questions that our conclusions raise:
- How significant are implicit redistributive mechanisms such as limited intergenerational transfers of wealth, and do they disproportionately affect wealthy households?
- Given that the extreme divergence in our model is driven largely by uninsurable idiosyncratic investment risk, how can this risk best be addressed by policymakers?
Finally, several questions about the role of explicit redistributive mechanisms such as government tax and fiscal policies arise. Our research suggests a role for such policies as a force that counters the natural tendency of wealth to concentrate. So, the final question is:
References
Atkinson, AB, T Piketty, and E Saez (2011), “Top incomes in the long run of history”, Journal of Economic Literature, 49(1): 3–71.
Benhabib, J, A Bisin, and S Zhu (2011), “The distribution of wealth and fiscal policy in economies with infinitely lived agents”, Econometrica, 79(1):123–57.
Cagetti, M and M De Nardi (2008), “Wealth inequality: Data and models”, Macroeconomic Dynamics, 12(S2): 285–313.
Case, KE and RJ Shiller (1989), “The efficiency of the market for single-family homes”, American Economic Review, 79(1): 125–137.
Davies, JB, S Sandström, A Shorrocks, and EN Wolff (2011), “The level and distribution of global household wealth”, Economic Journal, 121(551): 223–54.
Díaz-Giménez, J, V Quadrini, J-V Ríos-Rull, and SB Rodríguez (2002), “Updated facts on the U.S. distributions of earnings, income, and wealth”, Federal Reserve bank of Minneapolis Quarterly Review, 26(3): 2–35.
Fernholz, ER (2002), Stochastic Portfolio Theory, Springer-Verlag.
Fernholz, R and R Fernholz (2012), “Wealth distribution without redistribution”, Claremont McKenna College Working Paper.
Flavin, M and T Yamashita (2002), “Owner-occupied housing and the composition of the household portfolio”, American Economic Review, 92(1):345–62.
Gabaix, X (2009), “Power laws in economics and finance”, Annual Review of Economics, 1(1): 255–94.
Moskowitz, TJ and A Vissing-Jorgensen (2002), “The returns to entrepreneurial investment: A private equity premium puzzle?”, American Economic Review 92(4): 745–78.
Wolff, EN (2012), “Changes in household wealth in the 1980s and 1990s in the US”, in EN Wolff (ed), International Perspectives on Household Wealth, Cheltenham: Edward Elgar.